摘要:
本文建立了热传导方程的奇异内边界问题：求{u(x,t),x(t)}，
∂u/∂t=a2∂2u/∂x2-b∂u/∂x-ru+γ(t)δ(x-x(t)),-∞<x<+∞,t>0
u(x,0)=0,-∞<x<+∞
u(x(t),t)=max-∞<x<+∞ u(x,t)=φ(t),t≥0
∂u/∂t(x(t),t)=o,t≥0
limx→-∞|u|<∞,limx→+∞|u|<∞
使其满足
(I)
其中φ(t)为待求函数。并获得奇异内边界的线性函数表达式x(t)=x0+bt ，且解函数u(x,t) 满足u(x(t),t)=max-∞<x<+∞ u(x,t) 。同时获得了热传导方程的问题A (在区域-∞<x<x(t),t≥0上的自由边界问题)和问题B(在区域x(t)≤x<+∞,t≥0 上的自由边界问题)的自由边界皆为x(t)=x0+bt，问题A和问题B的自由边界与奇异内边界重合；线性函数表达式x(t)=x0+bt 为最佳热源位置边界。完全类似地，我们建立了Black-Scholes方程的奇异内边界问题：
求{u(s,t),s(t)},使其满足
∂u/∂t+(σ2/2)s2(∂2u/∂s2)+(r-q)s(∂u/∂s)-ru=-γ(t)δ(s-s(t)),0<s<+∞,0<t<T
u(s,T)=φ(s),0≤s<+∞
u(s(t),t)=φ(t),0<t<T
(∂u/∂s)(s(t),t)=v(t),0<t<T
lims→o+|u|<+∞,lims→+∞|u|<+∞
(II)
其中φ(t), v(t) 为待求函数。
获得：10 当终值函数φ=0且边值函数v=0时；奇异内边界为s(t)=sTeσ2ω(T-t) ，且解函数u(s,t)=w(s,t) 满w(s(t),t)=max0≤s<∞w(s,t) ; 20当终值函数 φ≠0时；获得奇异内边界为s(t)=sTeσ2ω(T-t) ，且解函数u(s,t)=v(s,t)+w(s,t) 满足w(s(t),t)=max0≤s<∞w(s,t) ，
v(t)=(∂v/∂s)(s(t),t),φ(t)=v(s(t),t)+w(s(t),t) 。同时建立了自由边界问题A(在区域0≤s≤s(t), (0,T) 上)和自由边界问题B(在区域s(t)≤s<+∞,(0,T)上)。获得问题A和问题B在齐次终值条件下确定的自由边界都为s(t)=sTeσ2ω(T-t) 。终值函数φ满足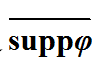
=[K,+∞) 或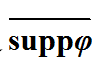=[0,K]得到 sT=K。从而问题A和问题B具有公共自由边界s(t)=Keθ(T-t)，满足条件(1/s(t))(ds(t)/dt)≡-θ ，常数θ=q-r+(1/2)σ2 由Black-Scholes方程中的参数q，r，σ2 唯一确定。
In this paper, the singular interior boundary problem of heat conduction equation is established:
seeking{u(x,t),x(t)} , satisfy
∂u/∂t=a2∂2u/∂x2-b∂u/∂x-ru+γ(t)δ(x-x(t)),-∞<x<+∞,t>0
u(x,0)=0,-∞<x<+∞
u(x(t),t)=max-∞<x<+∞u(x,t)=φ(t),t≥0
∂u/∂t(x(t),t)=o,t≥0
limx→-∞|u|<∞,limx→+∞|u|<∞
(I)
which φ(t) is the function to be determined.
And the linear function expression x(t)=x0+bt of singular inner boundary is obtained, satisfying u(x(t),t)=max-∞<x<+∞u(x,t). We establish free boundary problem A and free boundary problem B on homogeneous heat conduction equation.
The problem A is free boundary problem in region -∞<x<x(t),t≥0 . The problem B is free boundary problem in region x(t)≤x<+∞,t≥0 . It is obtained that free boundary about problem A and problem B are the linear function x(t)=x0+bt. The free boundary about problem A and problem B coincides with the singular inner boundary.
Similarly,we establish the singular interior boundary problem of Black-Scholes equation：
seeking{u(s,t),s(t)} , satisfy
∂u/∂t+(σ2/2)s2(∂2u/∂s2)+(r-q)s(∂u/∂s)-ru=-γ(t)δ(s-s(t)),-∞<x<+∞,t>0
u(s,T)=φ(s),0≤s<+∞
u(s(t),t)=φ(t),0<t<T
(∂u/∂s)(s(t),t)=v(t),0<t<T
lims→o+|u|<+∞,lims→+∞|u|<+∞
(II)
which φ(t) and v(t) are functions to be determined.
When final function φ=0 , and boundary value function v=0 , the singular inner boundary s(t)=sTeσ2ω(T-t) is obtained, and the solution function u(s,t)=w(s,t) satisfies w(s(t),t)=max0≤s<∞w(s,t) and when final function φ≠0 , the singular inner boundary s(t)=sTeσ2ω(T-t) is obtained, and the solution function u(s,t)=v(s,t)+w(s,t) satisfies w(s(t),t)=max0≤s<∞w(s,t) and boundary value function v(t)=(∂v/∂s)(s(t),t),φ(t)=v(s(t),t)+w(s(t),t) .
We establish free boundary problem A and free boundary problem B on homogeneous Black-Scholes equation.
The problem A is free boundary problem in region 0≤s≤s(t),(0,T) . The problem B is free boundary problem in region s(t)≤s<+∞,(0,T). It is determined s(t)=sTeσ2ω(T-t) that free boundary about problem A and problem B. The conclusion sT=K by the final value function φ(s) satisfies 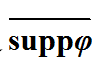=[K,+∞) or 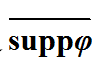=[0,K] . Thus the conclusion s(t)=Keθ(T-t) that is the public free boundary about the problem A and problem B,which satisfies the condition (1/s(t))(ds(t)/dt)≡-θ , constant θ=q-r+(1/2)σ2 determined by the parameters q，r，σ2 in the Black-Scholes equation.