一类障碍系统弱解的局部与全局高阶可积性
Local and Global Higher Integrability of Weak Solutions to a Class of Obstacle Systems
摘要:
本文讨论了一类偏微分方程的障碍系统 ,
,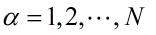 ,通过构造特殊的检验函数并利用逆Hölder不等式,得到了系统的弱解的局部和全局高阶可积性,从而把有关障碍问题
,通过构造特殊的检验函数并利用逆Hölder不等式,得到了系统的弱解的局部和全局高阶可积性,从而把有关障碍问题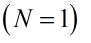 的一些结果推广到障碍系统
的一些结果推广到障碍系统  。
。
Abstract: This paper introduces obstacle systems for a class of quasilinear elliptic partial differential systems 
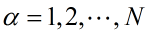 and obtains the local and global higher integrability of weak solutions to the obstacle systems by constructing special test functions and using Inverse Hölder’s Inequality. The results generalize some known results for obstacle problems
and obtains the local and global higher integrability of weak solutions to the obstacle systems by constructing special test functions and using Inverse Hölder’s Inequality. The results generalize some known results for obstacle problems 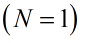 to obstacle systems
to obstacle systems  .
.
参考文献
|
[1]
|
A. Scott. Integrability, encyclopedia of nonlinear science. Taylor & Francis, 2005.
|
|
[2]
|
2G. Li, O. Martio. Local and global integrability of gradients in obstacle problems. Annales Academiae Scientiarum Fennicae Series A: Mathematica, 1994, 19(1): 25-34.
|
|
[3]
|
3E. N. Barron, R. Jensen. Minimizing the norm of the gradient with an energy constraint. Communications in Partial Differential Equations, 2005, 30(12): 1741-1772.
|
|
[4]
|
J. W. Cahn, C. A. Handwerker and J. E. Taylor. Geometric models of crystal growth. Acta Metallurgica, 1992, 40(7): 1443-1474.
|
|
[5]
|
J. Heinonen, T. Kilpelainen and O. Martio. Nonlinear potential theory of degenerate elliptic equations. New York: Clarendon Press, 1993.
|
|
[6]
|
M. Kubo, N. Yamazaki. Periodic solutions of elliptic-parabolic variational inequalities with time-dependent constraints. Journal of Evolution Equations, 2006, 6(1): 71-93.
|
|
[7]
|
G. Li, O. Martio. Stability of solutions of varying degenerate elliptic equations. Indiana Mathematics Journal, 1998, 47(3): 873-891.
|
|
[8]
|
M. Giaquinta, G. Modica. Regularity results for some classes of higher order nonlinear elliptic systems. Journal für die Reine und Angewandte Mathematik, 1979, 311-312: 145-169.
|
|
[9]
|
T. Iwaniec. The Gehring lemma. In: P. L. Duren, et al., Eds., Quasiconformal mappings and analysis: A collection of papers honoring Frederick W. Gehring on his 70th birthday. Ann Arbor: Proceedings of the International Symposium, August 1995, Berlin: Springer-Verlag, 1998: 181-204.
|
|
[10]
|
G. Li, O. Martio. Stability and higher integrability of derivatives of solutions in double obstacle problems. Journal of Mathematical Analysis and Applications, 2002, 272(1): 19-29.
|