一个N⊗+∞系统量子态的纠缠判据
An Entanglement Criterion for States in N⊗+∞System
DOI: 10.12677/AAM.2014.31003, PDF, HTML, 下载: 2,957  浏览: 12,280  国家自然科学基金支持
作者: 王银珠：太原理工大学数学学院，太原;太原科技大学数学系，太原
关键词: 无限维量子系统； 生成元；纠缠判据；Infinite Dimensional Quantum Systems; The Generators of ; Entanglement Criterion
摘要: 本文借助特殊酉群的生成元，研究了无限维两体量子态的可分性问题，得到了一些情形量子态可分的必要性判据(其中)。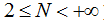
Abstract: In this paper, according to the generators of special unitary group , the separability of quantum states in infinite dimensional bipartite quantum systems is studied, and we obtain some necessary entanglement criteria for states in the cases of N⊗+∞().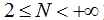

文章引用：王银珠. 一个N⊗+∞系统量子态的纠缠判据[J]. 应用数学进展, 2014, 3(1): 17-21. http://dx.doi.org/10.12677/AAM.2014.31003

参考文献

[1] Nielsen, M.A. and Chang, I.L. (2000) Quantum computation and quantum information. Cambridge University press, Cambridge.
[2] Horodecki, M., Horodecki, P. and Horodecki, R. (1996) Separablility of mixed states: necessary and sufficient conditions. Physics Letters A, 223, 1-14.
[3] Chen, K. and Wu, L.-A. (2003) A matrix realignment method for recognizing entanglement. Quantum Information & Computation, 3, 193-202.
[4] Rudolph, O. (2005) Further results on the cross norm criterion for separability. Quantum Information Processing, 4, 219-239.
[5] Nielsen, M.A. and Kempe, J. (2001) Separable states are more discorded globally than locally. Physical Review Letters, 86, 5184-5187.
[6] Zhao, H. and Wang, Z.X. (2004) Separable criterion for quantum mixed states. Theoretical Physics, 42, 529-534.
[7] Li, M., Fei, S.M. and Wang, Z.X. (2008) Separability of tripartite quantum systems. International Journal of Quantum Information, 6, 859-866.
[8] Holevo, A.S., Shirokov, M.E. and Werner, R.F. (2005) Separability and entanglement breaking in infinite dimensions. Russian Math Surveys, 60, 359-360.
[9] Hioe, F.T. and Eberly, J.H. (1981) N-level coherence vector and higher conservation laws in quantum optics and quantum mechanics. Physical Review Letters, 47, 838-841.
[10] Harrimann, J.E. (1978) Geometry of density matrices. I. Definitions, N matrices and 1 matrices. Physical Review A, 17, 1249-1256.

为你推荐