1. 引言
本文研究如下非线性抛物方程组解的爆破性
 (1.1)
(1.1)
 (1.2)
(1.2)
 (1.3)
(1.3)
 (1.4)
(1.4)
其中 是
是 上的有界区域且有光滑边界
上的有界区域且有光滑边界 ,
, ,
, ,
, 为以后给定的函数,
为以后给定的函数, 为正的初始函数。
为正的初始函数。
近几十年来,非线性抛物方程解的爆破问题引起人们的极大兴趣,考虑爆破性自然要研究解是否会爆破以及解的爆破时间 ,在这方面已经有大量的文献,如文献[1] -[5] 。问题(1.1)~(1.4)可以描述诸多化学反应、热传导过程和种群动力学过程(见文献 [6] - [17] 及其参考文献),它有多个非线性项,处理难度较大。对型如(1.1)的单个多重非线性抛物方程
,在这方面已经有大量的文献,如文献[1] -[5] 。问题(1.1)~(1.4)可以描述诸多化学反应、热传导过程和种群动力学过程(见文献 [6] - [17] 及其参考文献),它有多个非线性项,处理难度较大。对型如(1.1)的单个多重非线性抛物方程
 (1.5)
(1.5)
已有许多结果,如文献 [6] - [10] 给出了方程(1.5)的初边值问题和初值问题局部和整体解的存在性,文献 [11] - [14] 则研究了其整体吸引子的存在性和正则性,类似方程(1.1),(1.2)的方程组问题的整体吸引子的存在性和正则性也有一些研究 [15] - [17] 。但关于该类问题解的爆破性研究则相对较少,Iami和Mochizuki [18] 给出了Neumann初边值条件下方程(1.5)的爆破条件,Levine [19] - [21] 则用凸性方法证明了单个方程(1.1)及其等价的如下问题解的爆破性
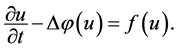
Korpusov和Sveshnikov [22] [23] 及Polat [24] 则对如下方程的初边值问题
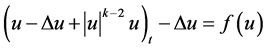
在负初始能量时得到解的爆破条件。Wang和Ge [25] 及其参考文献则利用比较原理讨论了非线性边界时方程(1.1),(1.2)中非线性项为 时解的爆破问题。
时解的爆破问题。
本文利用修正的Levine凸性方法讨论问题(1.1)~(1.4)解的爆破条件,推广了文献 [22] - [25] 的结果。在第二节将给出一些假设和基本引理,第三节给出主要结果和证明。
2. 假设和引理
本文所用符号均同文献 [6] ,记 和
和 为通常的Soblev空间,其范数分别记为
为通常的Soblev空间,其范数分别记为 和
和 ,特别是当
,特别是当 时,记
时,记 。
。
本文始终假设 。关于非线性项
。关于非线性项 的假设如下:
的假设如下:
(A1) ,存在函数
,存在函数 使得
使得
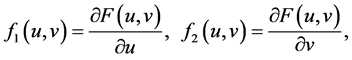
且存在常数 使得
使得
 ,
,
其中, 当
当 时,
时, 当
当 时。
时。
注:满足条件(A1)的函数是存在的。事实上,一个典型的例子是取
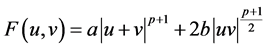
且 ,即
,即
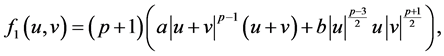
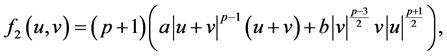
这时,  ,其中
,其中 ,
, 。该例的详细情况可见文献 [26] 。
。该例的详细情况可见文献 [26] 。
利用Galerkin方法,结合单调性理论和紧性方法 [2] ,类似文献 [27] 可得问题(1.1)~(1.4)解的局部存在性。
定理2.1. 假设条件(A1)成立且 ,
, ,
, ,则问题(1.1)~(1.4)存在弱解
,则问题(1.1)~(1.4)存在弱解 ,即,存在
,即,存在 使得
使得
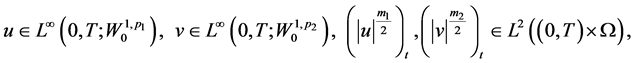
且对任意 和任意分别的
和任意分别的 (或
(或 )成立:
)成立:
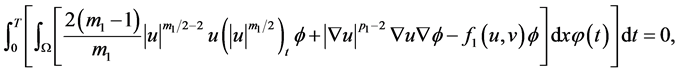
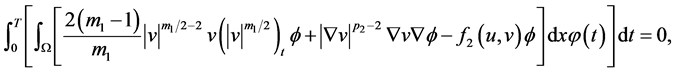
以及 。
。
下面给出本文的基本引理。
引理2.2. [5] [27] 设 是R上非负二次连续可导函数且满足不等式
是R上非负二次连续可导函数且满足不等式
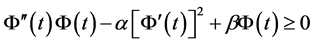
其中 为常数。若
为常数。若 ,
, ,则必存在时刻
,则必存在时刻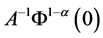
 ,使当
,使当 时有
时有 ,其中
,其中
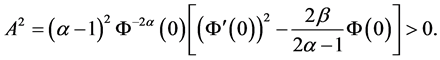
3. 主要结果及证明
首先引入泛函
 (3.1)
(3.1)
 (3.2)
(3.2)
 (3.3)
(3.3)
 (3.4)
(3.4)
现给出主要引理。
引理3.1. 对任意 ,下面不等式成立
,下面不等式成立
 (3.5)
(3.5)
证明 注意到
 (3.6)
(3.6)
注意到由Holder不等式得下列不等式
 (3.7)
(3.7)
 (3.8)
(3.8)
考虑到(3.7)~(3.8),则由(3.6)得
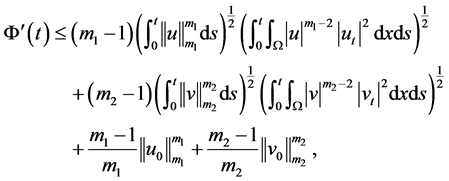
再利用不等式
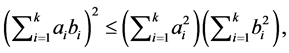
得
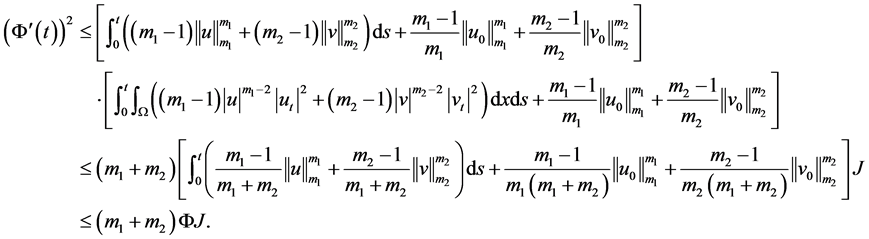
于是,引理得证。
下面,给出主要定理。
定理3.2. 设定理2.1的条件成立,且
 (3.9)
(3.9)
则问题(1.1)~(1.4)的弱解 必在某有限时刻
必在某有限时刻 爆破,即
爆破,即
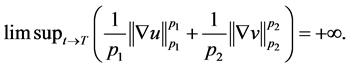
证明 注意到
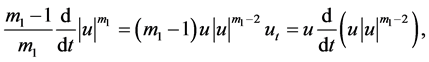
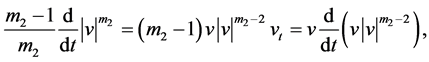
由(3.1),得
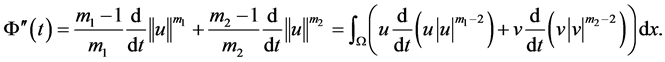
把(1.1),(1.2)代入得
 (3.10)
(3.10)
利用
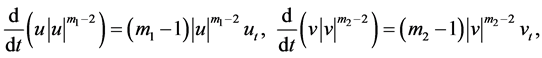
由(3.2)及方程(1.1),(1.2)得
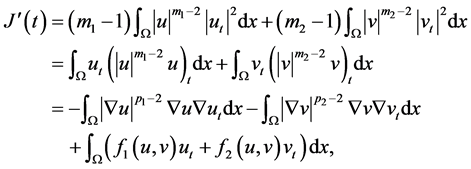
即
 (3.11)
(3.11)
(3.11)关于t积分得
 (3.12)
(3.12)
再利用条件(A1)得
 (3.13)
(3.13)
(3.10)结合(3.13),并用到 得
得
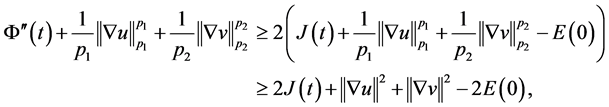
即
 , (3.14)
, (3.14)
注意到 得
得
 (3.15)
(3.15)
利用引理3.1,得

其中 。
。
如果 ,由(3.1),(3.6)和(3.9)知
,由(3.1),(3.6)和(3.9)知 ,
, ,于是,由引理2.2得结论。如果
,于是,由引理2.2得结论。如果 ,取
,取 ,则(3.15)变为
,则(3.15)变为
 (3.16)
(3.16)
于是,由(3.16)和标准的凸性引理得结论。综合两种情况即得 。
。