富勒烯C_10n的化学拓扑指数计算

Calculation of Topological Index for Fullerene C_10n

摘要: 在计算化学中，分子结构用图模型来表示称为分子图。其中每个顶点代表一个原子，每条边代表原子之间的化学键。研究发现，定义在分子图上的拓扑指数能反映化合物或者药物的化学性质。本文利用化学结构分析和边划分的方法得到富勒烯C_10n的第二类ABC指数，第二类GA指数，以及修改的Szeged指数。

Abstract: In computational chemistry, the molecular structures are modelled as graphs which are called the molecular graphs. In these graphs, each vertex represents an atom and each edge denotes covalent bound between atoms. It is shown that the topological indices defined on the molecular graphs can reflect the chemical characteristics of chemical compounds and drugs. In this paper, we present the second ABC index, the second GA index and modified Szeged index of fullerenes C_10n by means of chemical structure analysis and edge dividing techniques.

1. 引言

随着化学、制药学和材料科学中新技术的发展，每年都有大量的新化合物、新药物和新材料诞生。对这些新新化合物、新药物和新材料的生物化学特性进行测定变成一个复杂而繁琐的工作。在东南亚、非洲和拉丁美洲等欠发达地区，由于缺少购买设备和试剂的资金，使得对新药物和材料的测试工作成为无法完成的遗憾。然而，前期的大量化学实验发现，化学物质的性质与其分子结构的特征有着必然的联系。进而，在化学结构上的一些指标被定义出来，用于测定化学物质的特性(比如：熔点、沸点、毒性等等)。这项技术得到化学、制药、材料等领域的广泛关注。通过对特定化学分子结构进行某种指标的计算，可以了解其化学和药性特征(相关文献可参考[1] -[10] )。

在具体计算模型中，化学分子结构用图结构来表示：原子用顶点来表示，原子之间的化学健用边来表示。而定义在化学分子结构上的指数可以理解为一种映射，它将每个分子图G映射为一个(正)实数。在下文中，设G是一个化学分子图，设e = uv是图G中的一条边，代表在G中，离u顶点比离v顶点近的所有顶点的个数。同理，代表在G中，离v顶点比离u顶点近的所有顶点的个数。再设表示到u顶点的距离等于到v顶点的距离的顶点的个数。

Graovac和Ghorbani在文献 [11] 中定义了第二类化学键连通指数(the second atom-bond connectivity index，简称第二类ABC指数)如下：

。

Rostami等 [12] 得到关于第二类ABC指数的上界。

Fath-Tabar等 [13] 定义了第二类几何算术指数(the second geometric-arithmetic index，简称为第二类GA指数)如下：

。

Zhan和Qiao [14] 研究了树结构的最大和最小第二类GA指数，并给出对应的极图。

而修改的Szeged指数则定义为：

。

Xing和Zhou [15] 给出了n个顶点的单圈图的修改Szeged指数的极值。Chen等 [16] 对维纳指数和修改Szeged指数的差值进行了分析。Dong等 [17] 得到一些特殊分子结构族类的修改Szeged指数。Faghani和Ashrafi [18] 给出计算修改Szeged指数的新公式。

富勒烯(C60)发现至今只有短短30年时间，由于其独特的结构和物理、化学性质，吸引了众多科学家的目光，因此在这30年中，使得C60化学得到了很大的发展。富勒烯(Fullerene)是一种碳的同素异形体。任何由碳一种元素组成，以球状，椭圆状，或管状结构存在的物质，都可以被叫做富勒烯。富勒烯与石墨结构类似，但石墨的结构中只有六元环，而富勒烯中可能存在五元环。

本文研究富勒烯的距离相关指数。本文的贡献主要体现在如下三个方面：1) 得到的第二类ABC指数计算公式；2) 得到的第二类算数几何指数计算公式；3) 得到的修改的Szeged指数的计算公式。

2. 主要结果

通过对富勒烯的结构进行分析，并计算每条边e = uv的和值。根据和的具体值，可将富勒烯的边集合划分成如下15个子集：

图1所示各类边的参数计算结果如表1所示。

Figure 1. The dividing of

图1. 富勒烯的边集分类

Table 1. The related data for each edge class in fullerene C10n

表1. 富勒烯C_10n各类边的相关数据

若n为奇数，则以黄色部分进行计算；若n为偶数，则以绿色部分进行计算

根据图1的分类以及表1的计算结果，得到关于富勒烯的第二类ABC指数，第二类GA指数，和修改的Szeged指数如下：

若n为奇数，则

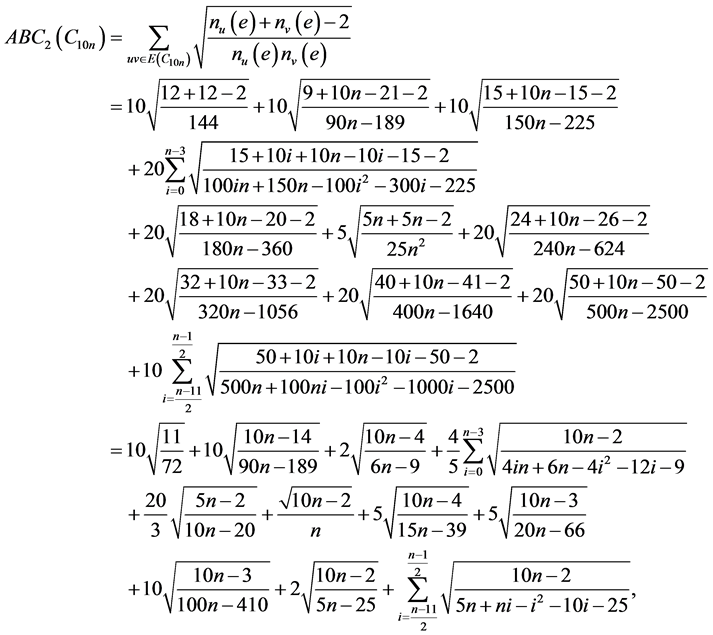
若n为偶数，则

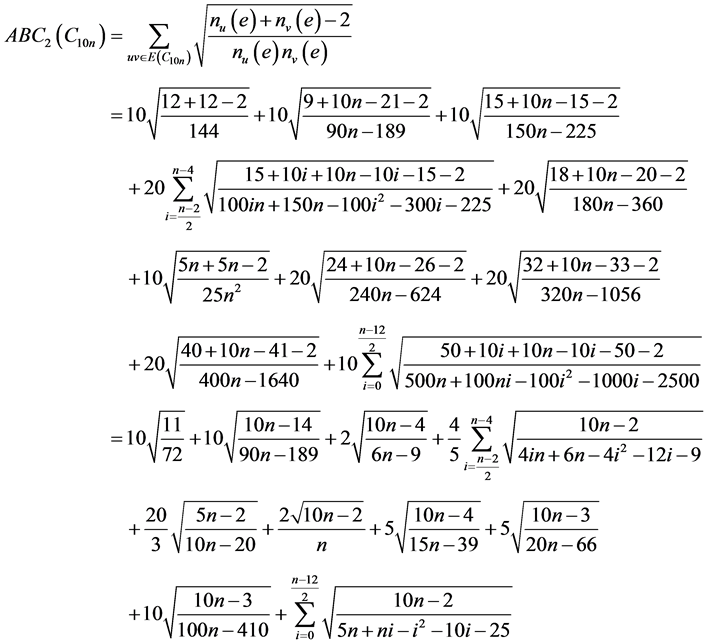
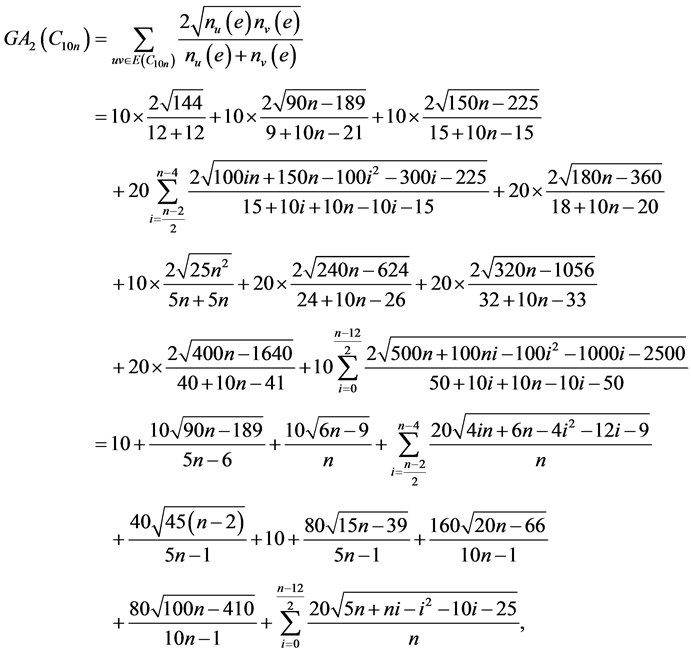
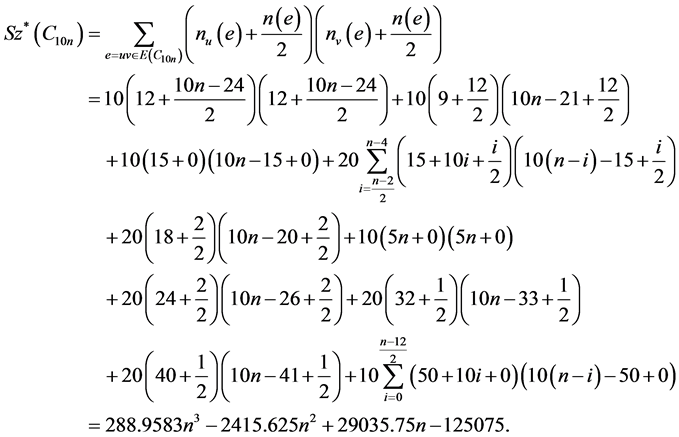
3. 总结

通过对富勒烯的化学结构进行分析，并计算每条边的，和值，对边集合进行分类，最后利用指数的定义给出具体表示公式。本文得到的计算结果对与富勒烯相关的化合物、材料和药物的分析具有理论指导作用。

基金项目

国家自然科学青年基金资助项目(11401519)。