摘要:
本文研究了形式三角矩阵环上Gorenstein AC-投射维数的问题。 令
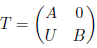
为一个形式三角矩阵环,其中A和B为环,U为一个(B,A)-双模,
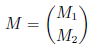
为左T - 模。 我们利用左A-模M
1和左B-模M
2的Gorenstein AC-投射维数,通过构造左T-模正合序列的方法给出了
TM的Gorenstein AC-投射维数的刻画,进而建立了环A,环B和环T的左整体Gorenstein AC-投射维数之间的关系。 作为这些结论的应用,我们刻画了环
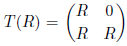
的左整体Gorenstein AC-投射维数及该环上左模的Gorenstein AC-投射维数。
Abstract:
This paper considers Gorenstein AC-projective dimensions over formal triangular matrix rings. Let
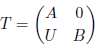
be a formal triangular matrix ring, where A and B are rings and U is a (B, A)-bimodule, and let

be a left T-module. By constructing exact sequences, we characterize Gorenstein AC-projective dimensions of a left T-module
TM with Gorenstein AC-projective dimensions of left A-module M
1 and left B-module M
2. Moreover, we establish a relationship of left global Gorenstein AC-projective dimensions of ring T and A, B. As an application of above conclusions, left global Gorenstein AC-projective dimension of the ring
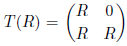
and Gorenstein AC-projective dimension of the left T(R)-module are described.