1. 引言
电磁规范理论本身包含物质相互作用的丰富内涵,它也具有启发产生各种新物理思想的很强的引领力量,曾在历史上引导物理学家创建相对论和量子力学,是优秀理论的典范[1] [2] 。本文探究了与电磁学有关的两个基本问题(电磁规范场和电磁真空能)对新的引力规范理论的启示意义。电磁规范理论及其推广(Yang-Mills理论)在量子场论和粒子物理领域取得了巨大成功要求引力理论也应当是某种局域规范对称下的规范场论;对于电磁真空能巨大引力效应与宇宙学观察事实之间的矛盾等问题的探究表明传统引力理论可能是一个低能唯象理论,可能还存在新的高能引力相互作用基本理论(这里所指的新的高能引力相互作用基本理论并非是指超对称引力与超弦理论那种从根本上革新广义相对论及其时空涵义的理论,而是指在这些“超”理论与爱因斯坦引力理论之间还存在一个基本的引力理论)。广义相对论引力理论存在三个基本问题(或明显不自洽的地方):① 理论数学结构与Yang-Mills规范场不同。在四种基本相互作用(强、弱、电、引力)中,前三种都是Yang-Mills规范场。唯独引力理论,虽然也有规范场特点,但其场方程与Yang-Mills规范场方程迥然不同;② 广义相对论引力理论存在带有量纲的引力常数,而Yang-Mills规范场不带有具有量纲的耦合系数;③ 广义相对论引力理论无法解释为何近乎无穷大密度的量子真空能体现不出引力效应。虽然量子真空能的负压确实能导致宇宙加速膨胀,1998年之后观察宇宙学(observational cosmology)确实发现宇宙加速膨胀的迹象,但它显然不能用量子真空能宇宙常数项来解释,因为量子真空能所导致的膨胀加速度将比实际观察到的大120个数量级。
本文介绍了一个新的自旋联络引力规范理论[3] 。它以局域Lorentz群为规范对称群、以自旋仿射联络作为非Abel规范场。该理论基于以爱因斯坦引力与Yang-Mills规范理论为线索。为了消去有量纲的引力常数,引入了一种重中介场(heavy intermediate field),它与物质场能耦合。该重中介场的质量很大,大约为普朗克质量量级。在低能条件下,我们可以获得一个度规的三阶微分方程作为引力场方程,而爱因斯坦引力场方程作为其首次积分解呈现。在该理论中,量子真空能巨大引力效应不再存在,并产生了一个新的宇宙学常数(作为首次积分解的积分常数),这可能为解决宇宙学常数之谜提供了一条思路[3] 。
我们还讨论了该引力规范理论的宇宙学意义。从该引力规范理论,我们可以得到一种准流体(quasi-fluid “matter state”),它本质上属于引力效应,但在四维宇宙中具有暗辐射(热暗物质)的特性;在五维宇宙中,可以表现为更为丰富的特性(如各向异性压强等)。结合该引力理论,我们提出了一个五维宇宙模型,把准流体当作引力源,可以发现准流体与第五维度可以提供某些暗物质与暗能量效应。在不需要引入真实的暗物质与暗能量(假如存在)的条件下,它就能产生等效暗物质与暗能量效应,它能自动解释为什么暗能量密度比零压物质(主要是暗物质)多两倍等宇宙学问题。
作为一篇综述性质的文章,本文先介绍规范理论历史来由与发展、量子真空能引力效应研究现状,然后引入一种我们建立的引力规范理论[3] ,对其引力作用量、低能近似与场方程作了研究,并探讨其在宇宙学中的意义。
2. 规范理论
Maxwell电磁理论为人类生产生活、改造自然与发展文明做出了伟大贡献。除了从功利角度看问题外,人们还发现Maxwell方程形式优美精巧雅致,是一件难得的艺术珍品,我们可以从多角度(代数、几何、拓扑等)来欣赏、把玩其美学价值。正是因为其理论中所包含的博大精深境界,电磁理论在历史上曾引导物理学家创建了近代物理两大支柱――相对论和量子力学。1865年Maxwell提出系统的电磁理论、创立了经典电动力学,尽管其理论比相对论的诞生早了40年,比量子力学完整框架的提出更早了60年,但可以毫不夸张地说,Maxwell电动力学是第一个相对论,也是第一个量子力学理论[1] [2] 。例如,Maxwell方程组天生具有Lorentz协变性,对其体系的考察使得一些物理学家如Poncaré,Lorentz和Einstein等人在1900年代初期革新了传统的牛顿力学,创建了相对论力学;Maxwell电动力学作为光的波动理论,其方程组属一次量子化理论范畴。就场论角度看,它是单粒子的量子场论(但因为光子不需要满足泡利不相容原理,这使得Maxwell方程看起来像是多粒子的场论),其与Schrödinger方程、Klein-Gordon方程和Dirac方程地位等价(它们都是单粒子的量子场论)[4] [5] 。作为经典场方程,Maxwell方程在进入量子场论之后,其基本形式仍旧保持(如Lagrangian密度仍旧被保留),这不免令人惊叹。此外,电磁理论在进入20世纪于量子力学和量子场论创立之时有两个立即的成果:① 规范理论的建立;② 电磁真空零点涨落能的发现。这大大丰富了我们对电磁场和电磁相互作用的认识,而且对研究其它基本相互作用也有诸多启示[1] [2] 。
在1918年,在当时爱因斯坦的“统一场论”指导思想激励下,Weyl提出了一种几何变换不变性(称作“规范变换不变性”),企图把电磁相互作用理论纳入引力理论(广义相对论)或者将电磁理论与引力理论统一起来[4] [5] 。但这种变换理论有一个明显的缺陷,即标度(尺子的长度)将依赖于其路径历史,这似乎与经验相悖。1925~1926年量子力学建立之后,人们发现带电粒子的量子力学波函数满足一种相位变换不变性,它与Weyl的几何变换不变性(“规范变换不变性”)的数学结构几乎一样,差别仅仅在于:前者(量子力学波函数)在指数变换因子上多了一个虚数单位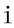 ,如此一来“标度”(尺子长度)将不再依赖于其路径历史(因为带虚数的指数变换因子形如
,如此一来“标度”(尺子长度)将不再依赖于其路径历史(因为带虚数的指数变换因子形如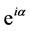 的模的平方为1)。实际上,这样一来,此时的“标度(尺子)”因子其实应该称为相位因子才对。所以,这种变换其实是相位变换,所谓的“不变性”,其实是“相位变换不变性”。但因为1929年Weyl基于以上思想在量子力学框架内重新表述他在1918年的工作时,对“相位变换”仍旧沿用“规范变换”一词,导致这一不恰当名词一直沿袭至今,四维电磁势
的模的平方为1)。实际上,这样一来,此时的“标度(尺子)”因子其实应该称为相位因子才对。所以,这种变换其实是相位变换,所谓的“不变性”,其实是“相位变换不变性”。但因为1929年Weyl基于以上思想在量子力学框架内重新表述他在1918年的工作时,对“相位变换”仍旧沿用“规范变换”一词,导致这一不恰当名词一直沿袭至今,四维电磁势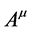 也就被称呼为规范场。因此可以这么说,对于电磁场本质和特性的进一步理解,受启发于爱因斯坦引力理论(广义坐标变换不变性,并在“统一场论”思想指导下欲图将它与广义相对论统一起来),但在量子力学建立之后,其规范场本质特性才完全凸显出来,这也使得荷电粒子的电动力学有更为精凑的形式[4] [5] 。在这之前,物理学家普遍认为在电磁学中真正有物理意义的是电磁场强度
也就被称呼为规范场。因此可以这么说,对于电磁场本质和特性的进一步理解,受启发于爱因斯坦引力理论(广义坐标变换不变性,并在“统一场论”思想指导下欲图将它与广义相对论统一起来),但在量子力学建立之后,其规范场本质特性才完全凸显出来,这也使得荷电粒子的电动力学有更为精凑的形式[4] [5] 。在这之前,物理学家普遍认为在电磁学中真正有物理意义的是电磁场强度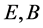 ,四维电磁势
,四维电磁势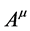 只是一个辅助量,不具有基本的物理意义。在解释Aharonov-Bohm效应时,第一次使得人们看到四维电磁势的物理含义。但在1954年之前,除了解释Aharonov-Bohm效应,电磁相互作用的规范理论一直产生不了进一步的较大的成果。看起来它是一个属于孤例的成果,不具有更深刻的基本理论意义。但在1954年,杨振宁和Mills迈出了重要的一步[4] -[6] 。受“质子和中子构成同位旋二重态”这一思想的启发,他们意图建立一套强相互作用的理论,仿照电动力学,将同位旋二重态作为一种荷,将四维电磁势推广到矩阵形式,建立了SU(2)非Abel规范理论,而原先的电磁理论在现在看来,其实是U(1) Abel规范理论。非Abel规范理论的“电磁势”因为是矩阵,故而彼此不再对易,这就导致非Abel规范理论的场方程是非线性的,因此非Abel规范场(称为Yang-Mills规范场)的量子具有自相互作用,比Maxwell电磁理论要复杂许多。基于Yang-Mills规范理论,上个世纪六七十年代建立了弱电统一理论和量子色动力学(两者构成了粒子物理的标准模型),并在1974年提出了大统一理论,企图把弱电统一理论和量子色动力学统一起来,即欲在Yang-Mills规范理论框架下将强、弱、电三种基本相互作用合并成一种基本力[4] [5] [7] 。弱电统一理论和量子色动力学取得了成功,它们的媒介子(规范粒子)即传递弱电相互作用的中间玻色子和传递强相互作用力的胶子都在实验上被发现了(或显示了迹象)[8] [9] 。
只是一个辅助量,不具有基本的物理意义。在解释Aharonov-Bohm效应时,第一次使得人们看到四维电磁势的物理含义。但在1954年之前,除了解释Aharonov-Bohm效应,电磁相互作用的规范理论一直产生不了进一步的较大的成果。看起来它是一个属于孤例的成果,不具有更深刻的基本理论意义。但在1954年,杨振宁和Mills迈出了重要的一步[4] -[6] 。受“质子和中子构成同位旋二重态”这一思想的启发,他们意图建立一套强相互作用的理论,仿照电动力学,将同位旋二重态作为一种荷,将四维电磁势推广到矩阵形式,建立了SU(2)非Abel规范理论,而原先的电磁理论在现在看来,其实是U(1) Abel规范理论。非Abel规范理论的“电磁势”因为是矩阵,故而彼此不再对易,这就导致非Abel规范理论的场方程是非线性的,因此非Abel规范场(称为Yang-Mills规范场)的量子具有自相互作用,比Maxwell电磁理论要复杂许多。基于Yang-Mills规范理论,上个世纪六七十年代建立了弱电统一理论和量子色动力学(两者构成了粒子物理的标准模型),并在1974年提出了大统一理论,企图把弱电统一理论和量子色动力学统一起来,即欲在Yang-Mills规范理论框架下将强、弱、电三种基本相互作用合并成一种基本力[4] [5] [7] 。弱电统一理论和量子色动力学取得了成功,它们的媒介子(规范粒子)即传递弱电相互作用的中间玻色子和传递强相互作用力的胶子都在实验上被发现了(或显示了迹象)[8] [9] 。
因此,自然界四种基本相互作用力的三种(强、弱、电)都属于Yang-Mills规范相互作用。还剩下引力相互作用,未被完全纳入到Yang-Mills规范理论框架。描述引力相互作用的成功理论是爱因斯坦广义相对论[10] [11] ,其属于广义坐标变换不变性的理论,与Yang-Mills规范理论的数学结构大为不同。根据研究相互作用的数学物理的某些基本规则和经验,引力理论也应当是一种Yang-Mills规范理论,虽然没有一个绝对令人信服的理由让人相信必须如此,但是,传统的引力理论的确暴露出诸多缺陷,强烈暗示爱因斯坦引力理论可能是一个低能唯象理论,在其背后可能还隐藏着一个更为基本的理论(说明:我们这里所谓的隐藏着的理论不是指局域超对称引力理论,也不是指弦理论。我们的意思是,即使在靠近Planck能标下,还存在着一个基本的引力理论,它必须是Yang-Mills型的)。一个恰当的比方是,如果把爱因斯坦引力理论看作是上个世纪三十年代的费米弱相互作用理论,那么新的引力规范理论类似于上个世纪六十年代所提出的格拉肖–温伯格–萨拉姆(Glashow-Weinberg-Salam)弱电统一理论, 而费米弱相互作用理论是它的低能唯象理论[4] [5] [7] 。
3. 量子真空能
量子力学和量子场论的建立使得我们对真空有了新的理解。真空不再是空无一物,它是场的基态,具有丰富的结构,呈现了多种效应,如真空极化,使得电子具有反常磁矩,也使得氢原子能级有兰姆移动、光谱有超精细结构[4] [5] [7] 。真空还具有零点涨落能,它使得原子激发态能级发生自发辐射衰变,使得真空中两块平行放置的金属板之间有微弱的Casimir力[12] 。真空具有零点涨落能是因为电磁场算符的不可对易性所造成的(电磁真空态不能同时是电场与磁场的本征态,因此电场与磁场都不能精确被测定)。对相空间进行积分或求和,人们发现量子真空能量密度是发散的。假使有截断能标,如Planck能标,这个真空能密度也是相当巨大的,大约是目前宇宙平均质量密度的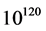 倍以上,即为宇宙视界尺度平方
倍以上,即为宇宙视界尺度平方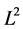 与Planck长度平方
与Planck长度平方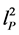 之比
之比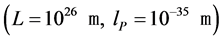 [13] -[18] 。该真空能密度由于均匀且各向同性,通常在自由空间的电磁学和量子光学领域并不表现可观测效应,除非电磁环境(电磁介质)均匀性和各向同性被破坏,如在金属微腔内、金属波导内、两面靠得极近的镜子之间,由于长波长量子真空模式被排挤,这会大大影响原子自发辐射衰变率[19] -[21] ;两块平板金属之间有微小吸引力,也是由于真空能所导致的Casimir效应[12] ;各向异性材料内的场量子化与各向同性介质内的场量子化最终结果不同,这使得各向异性材料内的真空能具有非零动量和角动量[22] -[26] 。此外,光子在非共面弯曲光纤内传播,也会呈现量子真空效应,如高孝纯首先研究了弯曲光纤内二次量子化光场的传播,并提出量子真空几何相因子的概念[27] (由于左右旋圆偏振真空模式的几何相位大小相等、符号相反,恰互相抵消,因而该真空相位无法直接体现出来,或许需要在各向异性介质内才有可能体现出来)。总之,在量子光学和电磁学中,量子真空零点涨落能要么表现不出效应,要么表现出来的效应比较微弱。但是对于引力领域,量子真空能由于具有巨大的能量密度,应该表现出非常强的引力效应,即使在局域惯性系中,它也应该具有非常大的引力落差效应,即潮汐力,使得任何基本粒子都撕得粉碎;对于宇宙尺度,量子真空能会加速膨胀宇宙(量子真空能密度本身与普通物质一样,呈现吸引效应,也使得宇宙收缩或塌缩,但量子真空能具有很大的负压,负压使得真空能表现为有斥力,具体说来,排斥力是吸引力的三倍,总的效果使得宇宙加速膨胀)。的确,在1998年之后,宇宙学家和天体物理学家的确观察到了宇宙的加速膨胀现象[28] -[30] ,但这显然不是由强大的量子真空能驱动的,因为假如宇宙的加速膨胀是由量子真空能驱动,那么只要大约占
[13] -[18] 。该真空能密度由于均匀且各向同性,通常在自由空间的电磁学和量子光学领域并不表现可观测效应,除非电磁环境(电磁介质)均匀性和各向同性被破坏,如在金属微腔内、金属波导内、两面靠得极近的镜子之间,由于长波长量子真空模式被排挤,这会大大影响原子自发辐射衰变率[19] -[21] ;两块平板金属之间有微小吸引力,也是由于真空能所导致的Casimir效应[12] ;各向异性材料内的场量子化与各向同性介质内的场量子化最终结果不同,这使得各向异性材料内的真空能具有非零动量和角动量[22] -[26] 。此外,光子在非共面弯曲光纤内传播,也会呈现量子真空效应,如高孝纯首先研究了弯曲光纤内二次量子化光场的传播,并提出量子真空几何相因子的概念[27] (由于左右旋圆偏振真空模式的几何相位大小相等、符号相反,恰互相抵消,因而该真空相位无法直接体现出来,或许需要在各向异性介质内才有可能体现出来)。总之,在量子光学和电磁学中,量子真空零点涨落能要么表现不出效应,要么表现出来的效应比较微弱。但是对于引力领域,量子真空能由于具有巨大的能量密度,应该表现出非常强的引力效应,即使在局域惯性系中,它也应该具有非常大的引力落差效应,即潮汐力,使得任何基本粒子都撕得粉碎;对于宇宙尺度,量子真空能会加速膨胀宇宙(量子真空能密度本身与普通物质一样,呈现吸引效应,也使得宇宙收缩或塌缩,但量子真空能具有很大的负压,负压使得真空能表现为有斥力,具体说来,排斥力是吸引力的三倍,总的效果使得宇宙加速膨胀)。的确,在1998年之后,宇宙学家和天体物理学家的确观察到了宇宙的加速膨胀现象[28] -[30] ,但这显然不是由强大的量子真空能驱动的,因为假如宇宙的加速膨胀是由量子真空能驱动,那么只要大约占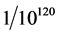 的量子真空能,就足以产生目前所能观察到的宇宙加速膨胀效应,而其它占
的量子真空能,就足以产生目前所能观察到的宇宙加速膨胀效应,而其它占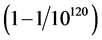 的量子真空能则没有表现出可观察的引力效应。
的量子真空能则没有表现出可观察的引力效应。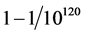 为
为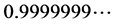 (共120个9),这本身已经说明,全部真空能其实并不贡献引力效应,而目前宇宙的加速膨胀必有其它根源,如目前传统观点认为,宇宙加速膨胀可能是由暗能量(精质quintessence 以及一些具有非寻常特性的phantom, quintom等)导致的[31] [32] 。那么具有巨大密度的量子真空能为何表现不出引力效应?这在目前广义相对论框架内是一个谜,且被认为是相当匪夷所思之谜,甚至可能是理论物理学中第一谜(最令人尴尬之谜)。这暗示要么我们对真空的量子场论需要重新理解,要么目前的引力理论需要重新审察。我们选择后一路线进行考察[3] 。在文献[3] 中所建立的引力规范理论,并非专为解决真空能宇宙常数问题而设。再将规范原理与引力结合起来,重新表述爱因斯坦引力之后,顺道替真空能宇宙常数问题提供了一个可能的解决思路。
(共120个9),这本身已经说明,全部真空能其实并不贡献引力效应,而目前宇宙的加速膨胀必有其它根源,如目前传统观点认为,宇宙加速膨胀可能是由暗能量(精质quintessence 以及一些具有非寻常特性的phantom, quintom等)导致的[31] [32] 。那么具有巨大密度的量子真空能为何表现不出引力效应?这在目前广义相对论框架内是一个谜,且被认为是相当匪夷所思之谜,甚至可能是理论物理学中第一谜(最令人尴尬之谜)。这暗示要么我们对真空的量子场论需要重新理解,要么目前的引力理论需要重新审察。我们选择后一路线进行考察[3] 。在文献[3] 中所建立的引力规范理论,并非专为解决真空能宇宙常数问题而设。再将规范原理与引力结合起来,重新表述爱因斯坦引力之后,顺道替真空能宇宙常数问题提供了一个可能的解决思路。
4. 广义相对论引力理论面临的问题以及出路
众所周知,不同作者从多种角度指出了爱因斯坦引力理论所面临的困难[33] -[50] 。这里只介绍其中几个与引力规范理论密切有关的问题[50] -[54] :
①爱因斯坦引力的规范理论形式贯彻不彻底。刻画时空的几何特性,有两个物理量,即曲率和挠率。从规范理论角度讲,黎曼曲率是局域齐次Lorentz规范协变下的规范场强,规范场是自旋仿射联络,其源是物质的自旋流密度张量;挠率,是局域时空平移(local spacetime translation)规范协变下的规范场强,规范场是度规或标架vierbein场,其源是物质的能量–动量张量。但在爱因斯坦引力理论中,这些都交织在一起,且局域规范不变思想显得不彻底[33] -[35] [50] [51] 。如其引力源是物质的能量–动量张量,但理论中并没有出现挠率;出现了黎曼曲率(作为规范场强),但理论中却不涉及物质源的自旋流密度张量;在Einsitein-Hilbert引力作用量中,基本动力学变量是度规或vierbein场,但整个理论的联络却是Christoffel符号(Levi-Civita对称联络),联络与动力学变量不是同一客体,这与Yang-Mills规范场论大为不同(在Yang-Mills理论中,联络与动力学变量是同一个物理量)。虽然之后Cartan等将挠率引入广义相对论,并将自旋作为挠率的源[46] -[49] ,但没有改变以上现状。
②引力常数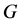 有
有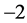 的质量量纲。这可能是造成引力理论不可重整化的原因之一(但在无物质源时,在引力作用量中添上度规的高阶导数项如曲率平方项等, 可以重整化)。我们希望一个基本的引力理论只含有无量纲的引力耦合系数。这也暗示爱因斯坦引力理论是一个低能唯象引力理论。
的质量量纲。这可能是造成引力理论不可重整化的原因之一(但在无物质源时,在引力作用量中添上度规的高阶导数项如曲率平方项等, 可以重整化)。我们希望一个基本的引力理论只含有无量纲的引力耦合系数。这也暗示爱因斯坦引力理论是一个低能唯象引力理论。
③宇宙学常数之谜。在爱因斯坦引力理论中,巨大的量子真空能密度应该呈现巨大的引力(以及潮汐力)。但实际上该巨大引力效应并没有被观察到(这在前面已经详细讨论过)[13] -[18] 。要是真的有该真空能的巨大引力呈现,那么即使在局域惯性系内时空任何一点都将有巨大的引力落差(潮汐力)存在,使得原子分子及原子核都将被引潮力撕裂。
为了遵照Yang-Mills规范理论精神及克服量子真空能引力效应问题,我们提出了一个自旋联络引力规范理论[3] [51] -[54] 。值得一提的是,在历史上,基于各种对称群以及诸多方案,各种引力规范理论不断有人提出,其中亦包括将自旋联络作为引力规范场的引力规范理论[33] -[44] 。但这些自旋联络引力规范理论中的Lagrange密度尽管包含了Riemann曲率平方项,实际上却仍旧是Hilbert-Einstein路线(方案)的拓展,因此并没有解决上节中的问题②和③。这里我们介绍一个新的自旋联络引力规范理论[3] ,它的低能情形包含了Stephenson-Kilmister-Yang引力理论[55] -[62] ,将爱因斯坦引力场方程作为一个首次积分解呈现出来。作为积分常数项,它还包含了一种准流体的能量-动量张量项(为准辐射场的能量-动量张量项和等效宇宙学常数项之和),因此可以作为暗物质和暗能量的可能候选者[63] 。不但如此,由于该自旋联络引力规范场方程为度规的三阶偏微分方程,真空能的能量-动量张量项实际以其协变导数形式出现,而因真空能的能量–动量张量的协变导数为零,故而真空能作为引力源,实际不能在该自旋联络引力规范场方程中显现,因此也就不体现任何引力效应[因为早已被“协变导数”(covariantly differentiated)掉了]。所以,在该自旋联络引力规范理论中,量子真空能引力效应问题便不再存在,也即在这个引力规范理论中,真空能宇宙学常数问题不再是一个疑难[3] 。
5. 自旋联络引力规范理论
在平直时空中,Maxwell方程为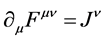 ,其中电磁场张量(规范场强、曲率)
,其中电磁场张量(规范场强、曲率) 
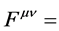
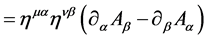 ,
,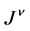 为电磁流密度。Maxwell方程的推广即Yang-Mills规范场方程为
为电磁流密度。Maxwell方程的推广即Yang-Mills规范场方程为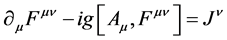 ,其中非Abel规范场强定义为
,其中非Abel规范场强定义为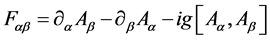 (
(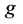 为无量纲的耦合系数)。由此受启发,对于引力场,以自旋仿射联络
为无量纲的耦合系数)。由此受启发,对于引力场,以自旋仿射联络 作为规范势[2] [31] ,引力规范场方程亦应是Yang-Mills形式:
作为规范势[2] [31] ,引力规范场方程亦应是Yang-Mills形式: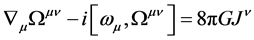 。 写出其全部分量,其完整方程是[3] [51]
。 写出其全部分量,其完整方程是[3] [51]
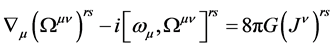 (1)
(1)
其流密度定义为:
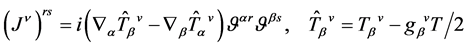 (2)
(2)
其中标量(由物质场的能量–动量张量缩并而得) 。值得顺便指出的是, 杨振宁提出一个杨氏引力场方程(无源情形)
。值得顺便指出的是, 杨振宁提出一个杨氏引力场方程(无源情形)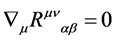 [55] -[62] 。在当
[55] -[62] 。在当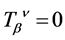 (无源情形)时[31] ,上述自旋联络引力规范场方程(1)即可以化为杨氏引力场方程[55] -[62] 。比杨氏理论更早,Stephenson和Kilmister也提出过该杨氏方程形式,故而杨氏理论也被称呼为Stephenson-Kilmister-Yang(SKY)引力理论[51] 。所以,SKY理论在本质上就是一个自旋联络引力规范理论[51] 。但是方程(1)给我们留下了不少问题:①其引力源
(无源情形)时[31] ,上述自旋联络引力规范场方程(1)即可以化为杨氏引力场方程[55] -[62] 。比杨氏理论更早,Stephenson和Kilmister也提出过该杨氏方程形式,故而杨氏理论也被称呼为Stephenson-Kilmister-Yang(SKY)引力理论[51] 。所以,SKY理论在本质上就是一个自旋联络引力规范理论[51] 。但是方程(1)给我们留下了不少问题:①其引力源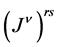 为何取形式(2)?②具有
为何取形式(2)?②具有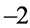 质量量纲的引力常数
质量量纲的引力常数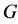 仍旧出现在方程(1)中,不甚令人满意;③其引力场Lagrange密度以及相互作用Lagrange密度取何种形式?
仍旧出现在方程(1)中,不甚令人满意;③其引力场Lagrange密度以及相互作用Lagrange密度取何种形式?
值得注意的是,上面对第①个问题中的引力源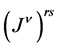 选取形式(2),乃是为了迎合或包容爱因斯坦引力场方程。所以我们需要通过对第③问题的回答即从基本原理出发来得到引力源
选取形式(2),乃是为了迎合或包容爱因斯坦引力场方程。所以我们需要通过对第③问题的回答即从基本原理出发来得到引力源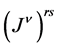 形式,同时希望把引力常数
形式,同时希望把引力常数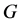 用适当方式消去,最终一举解决以上三个问题。在本例中,我们只研究以最简单的实标量场
用适当方式消去,最终一举解决以上三个问题。在本例中,我们只研究以最简单的实标量场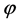 为引力源的情形。由于在自旋联络引力规范理论中,规范场张量即规范场强(实际上是Riemann曲率张量)的源是自旋流密度张量,但标量场是没有自旋流密度的,于是我们又引入一个重伴随场
为引力源的情形。由于在自旋联络引力规范理论中,规范场张量即规范场强(实际上是Riemann曲率张量)的源是自旋流密度张量,但标量场是没有自旋流密度的,于是我们又引入一个重伴随场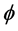 ,希望它起两个作用:①在低能(同时也含对经典场方程的量子修正)情形下,能提供一个等效的自旋流密度;②重伴随场
,希望它起两个作用:①在低能(同时也含对经典场方程的量子修正)情形下,能提供一个等效的自旋流密度;②重伴随场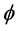 的重质量提供了对“引力常数
的重质量提供了对“引力常数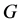 之所以微小”的解释。基于以上思路,新引力规范理论的引力场Lagrange密度为[3] [51] -[54]
之所以微小”的解释。基于以上思路,新引力规范理论的引力场Lagrange密度为[3] [51] -[54]
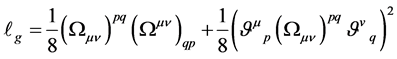 (3)
(3)
实标量场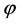 (物质场)和重伴随场
(物质场)和重伴随场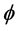 的Lagrange密度为
的Lagrange密度为
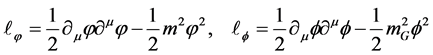 (4)
(4)
其相互作用Lagrange密度为
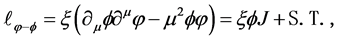 (5)
(5)
其中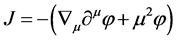 ,
,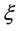 为新的无量纲引力耦合常数。 在路径积分方法中,
为新的无量纲引力耦合常数。 在路径积分方法中,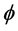 场关联函数的生成泛函为
场关联函数的生成泛函为
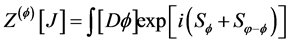 (6)
(6)
这里的作用量为
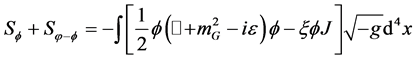 (7)
(7)
其中弯曲时空中的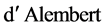 算子定义为
算子定义为
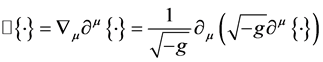 (8)
(8)
下面我们来考虑量子修正,把重中介场(heavy intermediate field)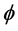 写为
写为 (如此,
(如此,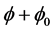 中的
中的 为经典场部分,
为经典场部分,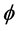 为量子修正)。令经典场
为量子修正)。令经典场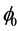 遵守经典场方程[3] [51] -[54]
遵守经典场方程[3] [51] -[54]
 (9)
(9)
其解为
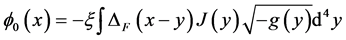 . (10)
. (10)
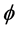 场的Green函数
场的Green函数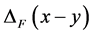 待求。利用式(10)以及
待求。利用式(10)以及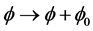 ,
,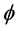 场的关联函数的生成泛函(6)成为[3]
场的关联函数的生成泛函(6)成为[3]
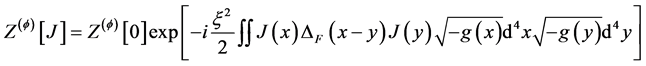 (11)
(11)
其中无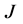 的生成泛函为
的生成泛函为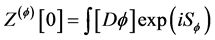 。在式(10)中,我们可以读出一个有效相互作用Lagrange密度(为非局域的形式)
。在式(10)中,我们可以读出一个有效相互作用Lagrange密度(为非局域的形式)
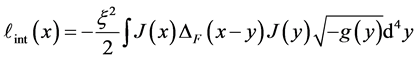 . (12)
. (12)
我们需要来计算(10)中的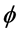 场的Green函数
场的Green函数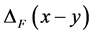 ,它满足方程
,它满足方程
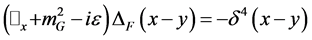 . (13)
. (13)
由于假设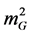 的绝对值很大,那么我们可以得到一个所感兴趣的低能近似解
的绝对值很大,那么我们可以得到一个所感兴趣的低能近似解
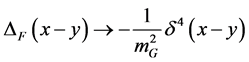 . (14)
. (14)
这样,非局域的有效相互作用Lagrange密度(12)为[3] [51] -[54]
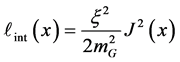 . (15)
. (15)
不妨令爱因斯坦引力系数 ,同时定义一个反对称张量
,同时定义一个反对称张量
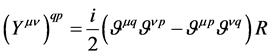 (16)
(16)
那么利用变分原理(或Euler-Lagrange方程),将自旋联络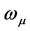 作为基本动力学变量,我们可以从Lagrange密度(3)(4)和(12)得到一个引力场方程[3] [51] -[54]
作为基本动力学变量,我们可以从Lagrange密度(3)(4)和(12)得到一个引力场方程[3] [51] -[54]
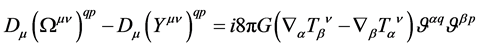 (17)
(17)
这里自旋联络协变导数定义为 。特别需要强调的是,这里
。特别需要强调的是,这里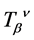 是物质场的emergent能量–动量张量[3] 。关于emergent 能量–动量张量的来源可见文献[3] 。(17)可以化为
是物质场的emergent能量–动量张量[3] 。关于emergent 能量–动量张量的来源可见文献[3] 。(17)可以化为
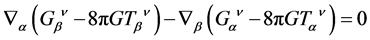 (18)
(18)
其中爱因斯坦张量为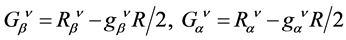 在这里出现。 我们发现方程(18)包含一个首次积分即
在这里出现。 我们发现方程(18)包含一个首次积分即

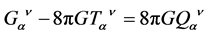 (19)
(19)
其中一个准物质(quasi matter)的能量–动量张量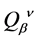 满足方程[53]
满足方程[53]
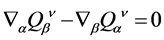 (20)
(20)
根据物质的能量–动量张量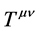 满足守恒定律
满足守恒定律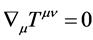 ,那么必然也要求准物质的能量–动量张量亦满足
,那么必然也要求准物质的能量–动量张量亦满足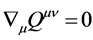 。再结合方程(20),我们可以证明
。再结合方程(20),我们可以证明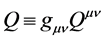 为常数,即
为常数,即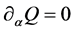 ,
,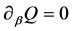 。对方程(19)进行缩并,可以得到
。对方程(19)进行缩并,可以得到
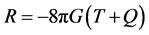 (21)
(21)
将此代入表达式(16),得到
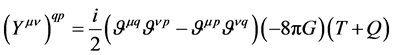 (22)
(22)
经过计算,其自旋联络协变导数为
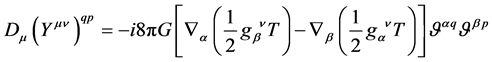 (23)
(23)
其中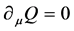 已经使用。再将式(23)代入自旋联络引力场方程(17),这样我们就得到了自旋联络引力规范场方程(1)。注意:自旋联络协变导数为
已经使用。再将式(23)代入自旋联络引力场方程(17),这样我们就得到了自旋联络引力规范场方程(1)。注意:自旋联络协变导数为 。这意味着该自旋联络引力规范理论可以得到SKY方程[55] -[62] ,SKY方程是该引力理论的一个低能有效理论。与此同时,我们得到了一个首次积分解,它是一个推广的爱因斯坦引力场方程(19),它包含了一个准物质的能量–动量张量
。这意味着该自旋联络引力规范理论可以得到SKY方程[55] -[62] ,SKY方程是该引力理论的一个低能有效理论。与此同时,我们得到了一个首次积分解,它是一个推广的爱因斯坦引力场方程(19),它包含了一个准物质的能量–动量张量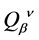 ,其满足方程(20)。方程(19)和(20)便成为新的自旋联络引力规范理论在低能情形下的引力场方程。显然可以看出,如果准物质的能量–动量张量
,其满足方程(20)。方程(19)和(20)便成为新的自旋联络引力规范理论在低能情形下的引力场方程。显然可以看出,如果准物质的能量–动量张量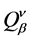 类似宇宙项,那么它必然满足方程(20),即该理论提供了一个新的等效的宇宙学常数。如果
类似宇宙项,那么它必然满足方程(20),即该理论提供了一个新的等效的宇宙学常数。如果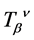 包含了真空能的能量–动量张量
包含了真空能的能量–动量张量 ,那么在三阶微分方程(18)中,因真空能的能量–动量张量
,那么在三阶微分方程(18)中,因真空能的能量–动量张量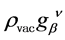 的协变导数自动为零,故而巨大的近乎发散的真空能将不在方程(18)中表现出引力效应。真空能发散引力问题便这样消去。
的协变导数自动为零,故而巨大的近乎发散的真空能将不在方程(18)中表现出引力效应。真空能发散引力问题便这样消去。
需要指出的是,标量物质场其实是没有自旋的,但是Lagrange密度(15)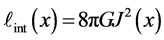 会导致一个等效“自旋流密度张量”
会导致一个等效“自旋流密度张量”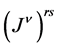 ,为自旋–联络引力规范场之源。方程(2)与(17)中的源
,为自旋–联络引力规范场之源。方程(2)与(17)中的源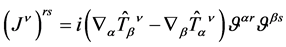 其性质类似等效“自旋流密度张量”(spin current density tensor),凡是自旋流密度张量具有的一般性质,它均有。
其性质类似等效“自旋流密度张量”(spin current density tensor),凡是自旋流密度张量具有的一般性质,它均有。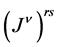 满足“等效自旋”守恒定律
满足“等效自旋”守恒定律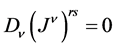 (这里,该守恒定律与另一个守恒定律
(这里,该守恒定律与另一个守恒定律 是一致的)。这说明“等效自旋”可以单独守恒,不必与轨道角动量一起才守恒。按照Einstein-Cartan理论的观念,这意味着物质场的能量–动量张量是对称的,理论不必引入挠率。但是,引力规范理论如果仅仅将自旋联络作为规范势是不够的,完备的引力规范理论还需要将度规或者vierbein作为满足时空平移规范对称的规范势、挠率是其规范场、能量–动量张量是其源(注意:这里所谓的“能量–动量张量”,是指Noether定理下的“能量–动量张量”,并非
是一致的)。这说明“等效自旋”可以单独守恒,不必与轨道角动量一起才守恒。按照Einstein-Cartan理论的观念,这意味着物质场的能量–动量张量是对称的,理论不必引入挠率。但是,引力规范理论如果仅仅将自旋联络作为规范势是不够的,完备的引力规范理论还需要将度规或者vierbein作为满足时空平移规范对称的规范势、挠率是其规范场、能量–动量张量是其源(注意:这里所谓的“能量–动量张量”,是指Noether定理下的“能量–动量张量”,并非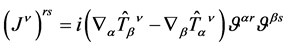 内的“能量–动量张量”。
内的“能量–动量张量”。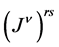 内的“能量–动量张量”是emergent“能量–动量张量”)。四维时空内的完备的引力规范理论包含的基本动力学变量(fundamental dynamical variable)是自旋仿射联络(共24个独立分量)与viernein(共16个独立分量),因此需要求Lagrange密度对自旋仿射联络与vierbein的变分(共得到40个方程)。由于自旋仿射联络直接与vierbein
内的“能量–动量张量”是emergent“能量–动量张量”)。四维时空内的完备的引力规范理论包含的基本动力学变量(fundamental dynamical variable)是自旋仿射联络(共24个独立分量)与viernein(共16个独立分量),因此需要求Lagrange密度对自旋仿射联络与vierbein的变分(共得到40个方程)。由于自旋仿射联络直接与vierbein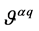 的导数与contortion(24个分量)密切相关,所以这类引力问题就在于求出vierbein与contortion的方程。由于认为等效自旋独立守恒
的导数与contortion(24个分量)密切相关,所以这类引力问题就在于求出vierbein与contortion的方程。由于认为等效自旋独立守恒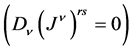 ,那么由16个vierbein方程,我们可以预测24个分量的contortion为零,也即挠率(torsion)为零。也就是我们仅仅由16个方程(vierbein方程)就输出了24个分量的信息。那么24个自旋联络方程就应该输出16个分量的vierbein
,那么由16个vierbein方程,我们可以预测24个分量的contortion为零,也即挠率(torsion)为零。也就是我们仅仅由16个方程(vierbein方程)就输出了24个分量的信息。那么24个自旋联络方程就应该输出16个分量的vierbein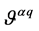 信息。自旋联络方程(具有24个分量)可以化为全微分形式,也就是具有首次积分解(vierbein的16个分量方程,即爱因斯坦方程)。以上仅对标量物质场情形成立。对于旋量和矢量物质场,情况就复杂多了,
信息。自旋联络方程(具有24个分量)可以化为全微分形式,也就是具有首次积分解(vierbein的16个分量方程,即爱因斯坦方程)。以上仅对标量物质场情形成立。对于旋量和矢量物质场,情况就复杂多了,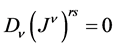 不再成立,因此必然需要挠率;自旋联络方程(具有24个分量)不再可以化为全微分形式,不再具有简单的首次积分解(爱因斯坦方程)。
不再成立,因此必然需要挠率;自旋联络方程(具有24个分量)不再可以化为全微分形式,不再具有简单的首次积分解(爱因斯坦方程)。
6. 对引力规范理论的讨论
求解准物质的能量–动量张量方程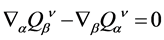 ,可以得到准流体(quasi fluid)
,可以得到准流体(quasi fluid)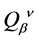 的表达式。在选取Robertson-Walker度规时,我们可以求得如下的精确解
的表达式。在选取Robertson-Walker度规时,我们可以求得如下的精确解
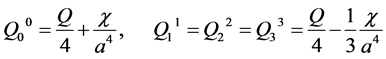 (24)
(24)
其余分量为零,其中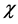 为实常数,
为实常数,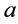 为宇宙标度因子。我们发现,
为宇宙标度因子。我们发现,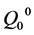 和
和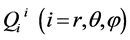 中的
中的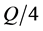 其地位就是宇宙常数
其地位就是宇宙常数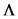 ,但是目前其物理意义不再是那个由量子真空零点涨落所导致的巨大(几近发散)的真空能量密度
,但是目前其物理意义不再是那个由量子真空零点涨落所导致的巨大(几近发散)的真空能量密度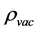 了,因为真空能的能量–动量张量
了,因为真空能的能量–动量张量 早在自旋联络引力场方程(17)和(18)中被“协变微分”(covariantly differentiated)掉了,因此不再呈现任何引力效应。但是,现在却出现了作为首次积分解的准流体能量–动量张量
早在自旋联络引力场方程(17)和(18)中被“协变微分”(covariantly differentiated)掉了,因此不再呈现任何引力效应。但是,现在却出现了作为首次积分解的准流体能量–动量张量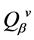 。它不但提供了一个宇宙学常数
。它不但提供了一个宇宙学常数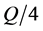 ,且还附带有一项准辐射(quasi radiation)的能量–动量张量(具有“暗辐射”特征)
,且还附带有一项准辐射(quasi radiation)的能量–动量张量(具有“暗辐射”特征)
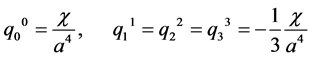 (25)
(25)
我们取流体的能量–动量张量为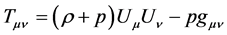 ,如果平直时空度规为
,如果平直时空度规为 ,则在随动坐标系且时空平直时,
,则在随动坐标系且时空平直时, ,
,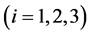
 ,我们有
,我们有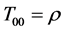 ,
,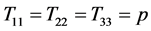 。而
。而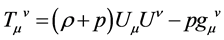 为
为
 (26)
(26)
因为其能量密度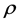 为
为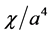 ,压强
,压强 为能量密度的三分之一,即
为能量密度的三分之一,即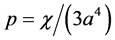 ,它们均与宇宙标度因子
,它们均与宇宙标度因子 的四次方成反比,因此具有辐射场的特征,但其不是真正的辐射型物质场,而是作为首次积分解而出现,故可名之为“准辐射”(quasi radiation)。我们认为,由于新的宇宙学常数
的四次方成反比,因此具有辐射场的特征,但其不是真正的辐射型物质场,而是作为首次积分解而出现,故可名之为“准辐射”(quasi radiation)。我们认为,由于新的宇宙学常数 和准辐射的出现,并考虑其宇宙学效应,将为我们解释暗能量和暗物质本质带来一条可能的思路。
和准辐射的出现,并考虑其宇宙学效应,将为我们解释暗能量和暗物质本质带来一条可能的思路。
标量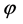 物质场、自旋联络引力场
物质场、自旋联络引力场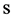 和重中介场
和重中介场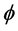 之间相互作用的典型Feynmann图可见图1。上节已经指出,尽管本理论不再追随Hilbert-Einstein路线,但仍旧可以得到爱因斯坦引力场方程(它作为该自旋联络引力规范理论的低能引力场方程的首次积分解形式出现),因此爱因斯坦引力理论可以看作是一个低能唯象引力理论。所谓低能情形,乃指
之间相互作用的典型Feynmann图可见图1。上节已经指出,尽管本理论不再追随Hilbert-Einstein路线,但仍旧可以得到爱因斯坦引力场方程(它作为该自旋联络引力规范理论的低能引力场方程的首次积分解形式出现),因此爱因斯坦引力理论可以看作是一个低能唯象引力理论。所谓低能情形,乃指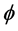 场作为中介粒子,其所传递的动量远远小于其静止质量
场作为中介粒子,其所传递的动量远远小于其静止质量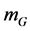 。根据爱因斯坦引力系数定义
。根据爱因斯坦引力系数定义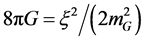 ,
,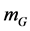 大约是
大约是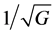 量级,所以
量级,所以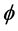 场的质量
场的质量 是极其巨大的(为Planck质量量级)。本文所得到的引力场方程(17)就是针对远低于Planck能量
是极其巨大的(为Planck质量量级)。本文所得到的引力场方程(17)就是针对远低于Planck能量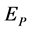 的情形,这也体现在
的情形,这也体现在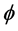 场的Green函数之中,即
场的Green函数之中,即 ,它使得非局域的有效相互作用Lagrange密度(12)变为了接触作用的Lagrange密度(15),可见图2(b)。
,它使得非局域的有效相互作用Lagrange密度(12)变为了接触作用的Lagrange密度(15),可见图2(b)。
在高能引力相互作用(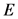 远大于
远大于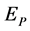 )中,比起相互作用特征时间
)中,比起相互作用特征时间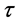 (约为
(约为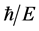 )来,中介场
)来,中介场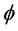 的寿命(约为
的寿命(约为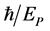 )远大于
)远大于 ,故而看起来中介场
,故而看起来中介场 有较长的寿命(在图2(a)中以粗线表示重粒子
有较长的寿命(在图2(a)中以粗线表示重粒子 );但在低能引力
);但在低能引力
 (a)
(a)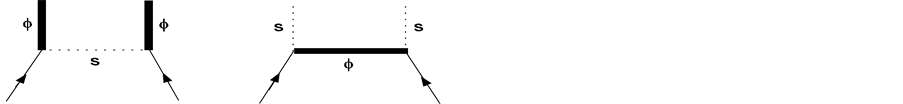 (b)
(b)
Figure 1. The Feynman diagrams of the scalar matter field φ, the spin-connection gravitational gauge field  and the heavy intermediate field f
and the heavy intermediate field f
图1. 标量φ物质场、自旋联络引力规范场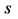 和重中介场f之间相互作用的典型Feynmann图
和重中介场f之间相互作用的典型Feynmann图
相互作用(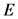 远小于
远小于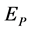 )中,比起相互作用特征时间
)中,比起相互作用特征时间 (约为
(约为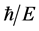 )来,中介场
)来,中介场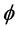 的寿命(约为
的寿命(约为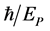 )远小于
)远小于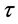 ,故而看起来中介场
,故而看起来中介场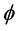 只有非常短的寿命或产生后即湮灭(在图2(b)中以相互作用顶角表示
只有非常短的寿命或产生后即湮灭(在图2(b)中以相互作用顶角表示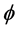 ),它无法从正面显示其全部动力学贡献,躲在背景中在效果上只提供了一个有量纲的引力常数
),它无法从正面显示其全部动力学贡献,躲在背景中在效果上只提供了一个有量纲的引力常数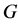 。
。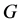 的表达式为
的表达式为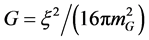 ,这就是引力常数的物理起源。在高能情形时,新的无量纲引力耦合常数
,这就是引力常数的物理起源。在高能情形时,新的无量纲引力耦合常数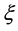 将代替有量纲的引力常数
将代替有量纲的引力常数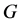 。此时,重粒子中介场
。此时,重粒子中介场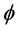 的动力学需要细致考虑。
的动力学需要细致考虑。
7. 引力规范理论的意义
上述引力理论[3] [51] -[54] 与SKY理论[55] -[62] 及爱因斯坦引力理论的关系是:上述引力规范理论(具有无量纲的引力常数)在低能近似下(远低于普朗克能标)的引力理论(呈现有量纲的引力常数以及允许具有可变宇宙学常数)的一个特例便是SKY理论(具有常数“宇宙学常数”),而SKY理论的一个特例(首次积分解)是爱因斯坦引力理论。由于SKY理论天然具有不变的宇宙学常数,故而SKY理论的真空解便是de Sitter时空[33] -[37] 。郭汉英等(在陆启铿早年建议下)一直倡导“de Sitter时空相对论”,放弃了狭义相对论对于刚尺服从欧氏几何(即零常曲率空间几何)的假定、改为让刚尺遵守具有非零常曲率空间的非欧几何,证明即使像de Sitter时空这样的弯曲时空内也有惯性参考系和惯性运动(而在广义相对论看来它仅有局域惯性系和局域惯性运动),并建立了局域de Sitter不变性的引力规范理论[36] [37] 。该理论的引力作用量密度为具有Yang-Mills形式的曲率平方项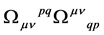 与挠率平方项
与挠率平方项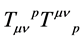 之和[36] 。但我们认为这里存在问题,因为将
之和[36] 。但我们认为这里存在问题,因为将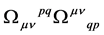 关于自旋联络求变分,可得到
关于自旋联络求变分,可得到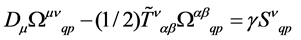 (方程右边是物质场的自旋流密度张量,
(方程右边是物质场的自旋流密度张量, 是与挠率有关的量)。进一步对方程左边求协变导数,得到
是与挠率有关的量)。进一步对方程左边求协变导数,得到 (其中
(其中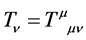 ),但是前述方程右边的自旋流密度张量
),但是前述方程右边的自旋流密度张量 的协变导数
的协变导数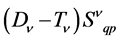 却不为零(因为自旋角动量并不单独守恒,要与轨道角动量一起才守恒),于是导致矛盾。但利用本文的引力作用量密度(3)就不会出现这个矛盾, 也就是说引力规范理论(最好能与Yang-Mills规范理论靠拢)其实不应该具有Yang-Mills形式的作用量密度。
却不为零(因为自旋角动量并不单独守恒,要与轨道角动量一起才守恒),于是导致矛盾。但利用本文的引力作用量密度(3)就不会出现这个矛盾, 也就是说引力规范理论(最好能与Yang-Mills规范理论靠拢)其实不应该具有Yang-Mills形式的作用量密度。
需要强调的是,上述引力理论[3] [51] -[54] 表明,牛顿-爱因斯坦引力其实是自旋联络规范理论中的引力效应的量子剩余,也即普通的牛顿-爱因斯坦引力本身就是一种量子效应[63] 。在高能Lagrangian密度(3)~(5)中,是不存在牛顿-爱因斯坦引力的。但在路径积分量子化过程中,通过计算物质场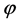 及重中介场
及重中介场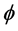 的关联函数生成泛函过程中,把物质场看作在经典场方程基础上的量子微挠,则可以得到Lagrangian密度的量子修正,即(15)式低能Lagrangian密度
的关联函数生成泛函过程中,把物质场看作在经典场方程基础上的量子微挠,则可以得到Lagrangian密度的量子修正,即(15)式低能Lagrangian密度 ,由它与引力Lagrangian密度(3)一起最终可以导致牛顿-爱因斯坦引力。
,由它与引力Lagrangian密度(3)一起最终可以导致牛顿-爱因斯坦引力。
本文所介绍的引力规范理论[3] [51] -[54] 可以解释一个看起来有点平凡(trivial)的问题,即为什么引力(gravity)是吸引力(attractive force)。此处的引力(系牛顿–爱因斯坦引力)乃是引力规范理论的低能近似现象(远低于普朗克能标,呈现有量纲的引力常数)。关于同种电荷之间的静电力为什么是斥力、质量之间的引力(gravity)为什么是吸引力(attractive force),传统的一种看法是:凡是交换自旋为1的矢量规范粒子(如光子)的相互作用表现为排斥力,凡是交换自旋为2的张量规范粒子(引力子)的相互作用表现为吸引力。实际上,对于前者(静电斥力),我们认为,这可还以从Lagrange量结构这一角度来解释(无论电磁协变导数 还是
还是 ,最终都表现为同种电荷之间的静电力为排斥力)。对于质量之间的引力为什么是吸引力,可以从上述(15)式低能Lagrangian密度
,最终都表现为同种电荷之间的静电力为排斥力)。对于质量之间的引力为什么是吸引力,可以从上述(15)式低能Lagrangian密度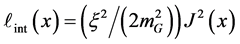 看出,即无论无量纲引力常数
看出,即无论无量纲引力常数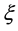 是正是负,最终的低能Lagrangian密度都是同号,且质量之间表现为吸引力,不会表现为排斥力。
是正是负,最终的低能Lagrangian密度都是同号,且质量之间表现为吸引力,不会表现为排斥力。
无穷大(或近乎发散的)量子真空能密度的引力效应问题可以算是当今理论物理学最令人惊异、尴尬的问题。由于量子真空能在引力理论中可以表现为一个宇宙常数项,因此量子真空能问题也被包含在宇宙学常数问题[13] -[15] 之中。随着引力理论和宇宙学的发展(尤其是自1998年起发现宇宙加速膨胀之后),宇宙学常数问题的表述也略有变化。一类表述是:为什么量子真空能没有表现为引力效应?为什么量子真空能宇宙常数近乎为零?为什么宇宙常数非零却很小(其密度与宇宙临界密度同量级)?如果量子真空能也是一种暗能量,为什么其密度与现在的宇宙临界密度具有相同数量级?宇宙学常数所对应的密度与现在的宇宙临界密度具有相同数量级,这个问题被称为宇宙学偶遇(一致)问题(cosmic coincidence problem)。 由于根据暴涨学说(早期宇宙的标度因子随时间呈指数膨胀),在宇宙学动力学方程(Friedmann方程)中的空间曲率项可以不计,因此现今宇宙物质 (暗能量、暗物质、普通重子物质)总密度也就等于宇宙临界质量密度,因此这个宇宙学偶遇(一致)问题也就表现为问:为什么宇宙学常数所对应的密度(或暗能量密度)与无压物质(暗物质、普通重子物质)密度具有相同数量级(密度比值基本上是3:1,更精确一点的比值是7:3)?总而言之,按照一般的观点,宇宙学常数问题可以分为两个部分:①细调问题(fine tuning problem),即如何通过精细调节,让无穷大(或近乎发散的)量子真空能密度被某负(裸)宇宙常数精密抵消到只剩下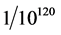 ;②宇宙密度的偶遇(一致)问题(cosmic coincidence problem) [13] -[15] 。
;②宇宙密度的偶遇(一致)问题(cosmic coincidence problem) [13] -[15] 。
为什么零压物质(暗物质与重子物质之和)密度与暗能量密度同数量级?这一问题所包含的内容是令人奇怪的,因为零压物质(暗物质与重子物质)与负压暗能量(或宇宙常数项)其实没有多大关系,随着宇宙膨胀其密度的演化规律也大不一样,如在零压物质或者冷暗物质为主时期,这些物质的密度随着宇宙年龄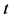 的演化规律为
的演化规律为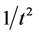 ;而在当今宇宙加速膨胀时期,暗物质与重子物质的密度下降将更快,演化规律大致为
;而在当今宇宙加速膨胀时期,暗物质与重子物质的密度下降将更快,演化规律大致为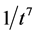 (设密度演化规律为
(设密度演化规律为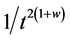 。根据目前ΛCDM模型,暗能量占总宇宙密度的七成,即大约5/7,因此平均物态系数为
。根据目前ΛCDM模型,暗能量占总宇宙密度的七成,即大约5/7,因此平均物态系数为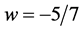 (平均物态系数是有物理含义的。根据减速参数的定义,
(平均物态系数是有物理含义的。根据减速参数的定义,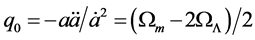 ,其中
,其中 和
和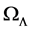 分别为零压物质密度和(宇宙常数所对应的)
分别为零压物质密度和(宇宙常数所对应的)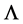 密度与宇宙临界密度的比值,它可以化为
密度与宇宙临界密度的比值,它可以化为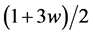 ,
,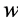 为平均物态系数)。将平均物态系数
为平均物态系数)。将平均物态系数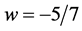 代入密度演化规律
代入密度演化规律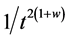 ,就可以得到零压物质密度演化规律大致为
,就可以得到零压物质密度演化规律大致为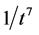 。当然,这规律仅对物质密度不是主要成份时适用。但是实际上目前零压物质还不可为极次要成份,又因为宇宙膨胀刚从减速过渡到加速,其演化规律不可能下降得比
。当然,这规律仅对物质密度不是主要成份时适用。但是实际上目前零压物质还不可为极次要成份,又因为宇宙膨胀刚从减速过渡到加速,其演化规律不可能下降得比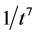 还要快,也可能大致为
还要快,也可能大致为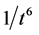 、
、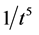 等。但无论如何,总之,零压物质密度还是下降得比较快的),而
等。但无论如何,总之,零压物质密度还是下降得比较快的),而 暗能量密度近乎不变。如果在零压物质与
暗能量密度近乎不变。如果在零压物质与 暗能量(负压)的密度两者之中,其中一方占压倒性的多数,则倒显得自然,这儿就不成为问题。可是在今天的宇宙中,它们的密度恰恰为同数量级(暗能量密度比零压物质多两倍,如接近3:1或7:3),这就成为一个令人困惑的疑问了,似乎我们处于一个比较特殊的宇宙演化时期。
暗能量(负压)的密度两者之中,其中一方占压倒性的多数,则倒显得自然,这儿就不成为问题。可是在今天的宇宙中,它们的密度恰恰为同数量级(暗能量密度比零压物质多两倍,如接近3:1或7:3),这就成为一个令人困惑的疑问了,似乎我们处于一个比较特殊的宇宙演化时期。
在本文所介绍的我们的引力规范理论[3] [51] -[54] 中,上述第①个问题其实已经被解决:在该引力规范场方程(为度规的三阶微分方程,爱因斯坦引力场方程系其首次积分解)中,量子真空能的能量–动量张量已经被“协变微分”掉了,因而无穷大的量子真空能密度表现不出其引力效应。对于第②个问题,虽然是一个疑难,但也可以大大被弱化。毕竟宇宙在过去曾经经历了一个很长时期的减速膨胀、零压物质密度下降的过程(密度演化规律为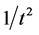 ),又兼现在既然有加速膨胀、暗能量密度逐渐占主导,物质密度占一定比例,但弱小,倒也可以算自然,也就是说,暗能量密度逐渐占主导,重子物质密度占一定比例、宇宙由减速膨胀、匀速膨胀、过渡到加速膨胀,这要占很长一段时期,因此我们处于该段时期的几率比较大(如果更早,星系还未演化出来;如果再晚,星系要被加速膨胀而瓦解,都不利于智慧生物生存)。因此,宇宙学密度之偶遇(一致)问题,虽令人困惑,倒也确实不一定必然是一个麻烦。值得一提的是,将本文所叙引力规范理论[3] [51] -[54] 应用到五维宇宙中去[63] ,并设准物质(quasi matter)为主要引力源(其能量–动量张量
),又兼现在既然有加速膨胀、暗能量密度逐渐占主导,物质密度占一定比例,但弱小,倒也可以算自然,也就是说,暗能量密度逐渐占主导,重子物质密度占一定比例、宇宙由减速膨胀、匀速膨胀、过渡到加速膨胀,这要占很长一段时期,因此我们处于该段时期的几率比较大(如果更早,星系还未演化出来;如果再晚,星系要被加速膨胀而瓦解,都不利于智慧生物生存)。因此,宇宙学密度之偶遇(一致)问题,虽令人困惑,倒也确实不一定必然是一个麻烦。值得一提的是,将本文所叙引力规范理论[3] [51] -[54] 应用到五维宇宙中去[63] ,并设准物质(quasi matter)为主要引力源(其能量–动量张量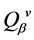 遵守公式(20)
遵守公式(20)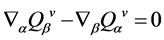 ),那么可以证明[63] ,五维宇宙中的额外维与准物质
),那么可以证明[63] ,五维宇宙中的额外维与准物质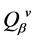 可以一起提供必要的等效暗物质与暗能量效应(相当于我们四维时空宇宙内的部分暗物质、暗能量效应其实来自于隐藏着的第五维空间的引力效应),其在四维宇宙中表现的平均物态系数
可以一起提供必要的等效暗物质与暗能量效应(相当于我们四维时空宇宙内的部分暗物质、暗能量效应其实来自于隐藏着的第五维空间的引力效应),其在四维宇宙中表现的平均物态系数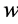 小于
小于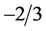 [63] ,如只要设准物质密度
[63] ,如只要设准物质密度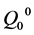 远大于普通重子物质密度(重子物质密度占宇宙临界密度的3%~5%),那么五维宇宙内的平均物态系数
远大于普通重子物质密度(重子物质密度占宇宙临界密度的3%~5%),那么五维宇宙内的平均物态系数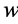 可以约为
可以约为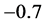 ,宇宙减速参数
,宇宙减速参数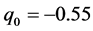 (实际数据可以结合当今哈勃参数或宇宙临界密度来定出)。根据1998年之后的精确宇宙学数据[28] -[30] ,当今宇宙诸主要组分中, 零压暗物质约占23%,可见的重子物质约占4%,负压暗能量约占73% (十余年前的数据)[64] ,则平均物态系数为
(实际数据可以结合当今哈勃参数或宇宙临界密度来定出)。根据1998年之后的精确宇宙学数据[28] -[30] ,当今宇宙诸主要组分中, 零压暗物质约占23%,可见的重子物质约占4%,负压暗能量约占73% (十余年前的数据)[64] ,则平均物态系数为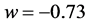 ,宇宙减速参数约为
,宇宙减速参数约为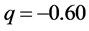 ;在最近几年的观察宇宙学中,根据进一步的模型改善与计算验证,一般认为当今宇宙诸主要组分中,暗物质约占26.5%,重子物质约占5%,暗能量约占68.5%[65] ,则平均物态系数为
;在最近几年的观察宇宙学中,根据进一步的模型改善与计算验证,一般认为当今宇宙诸主要组分中,暗物质约占26.5%,重子物质约占5%,暗能量约占68.5%[65] ,则平均物态系数为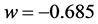 ,减速系数是
,减速系数是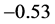 。因此,我们认为将引力规范理论与五维宇宙模型结合,借助当今哈勃参数(或宇宙临界密度)数据,可以得到与观察一致的宇宙减速参数。我们这个模型[63] 的优点是,不需要引入额外的暗物质与暗能量,它就能产生等效暗物质与暗能量效应,它自动解释了为什么暗能量密度比零压物质多两倍,如接近3:1或7:3。也就是说,我们可以解决上述第②个问题(cosmic coincidence problem),或者至少大大弱化该问题。为得到上述五维宇宙模型[63] 的结论(如平均物态系数
。因此,我们认为将引力规范理论与五维宇宙模型结合,借助当今哈勃参数(或宇宙临界密度)数据,可以得到与观察一致的宇宙减速参数。我们这个模型[63] 的优点是,不需要引入额外的暗物质与暗能量,它就能产生等效暗物质与暗能量效应,它自动解释了为什么暗能量密度比零压物质多两倍,如接近3:1或7:3。也就是说,我们可以解决上述第②个问题(cosmic coincidence problem),或者至少大大弱化该问题。为得到上述五维宇宙模型[63] 的结论(如平均物态系数 小于
小于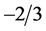 )的一个前提是需要假设准物质(quasi matter)为主要引力源(取这个假设的另外一个原因是只有单一物态存在时才可以得到方程(20)
)的一个前提是需要假设准物质(quasi matter)为主要引力源(取这个假设的另外一个原因是只有单一物态存在时才可以得到方程(20) 及五维宇宙的动力学方程(Friedmann方程)的精确解析解, 也即这是一个“工作假设”)。如果真的要完全回答宇宙物质密度的一致性问题(cosmic coincidence problem),那么就需要解释为什么“五维宇宙内准物质(quasi matter)为主要引力源”。作为一个观察事实(当今宇宙平均物态系数为
及五维宇宙的动力学方程(Friedmann方程)的精确解析解, 也即这是一个“工作假设”)。如果真的要完全回答宇宙物质密度的一致性问题(cosmic coincidence problem),那么就需要解释为什么“五维宇宙内准物质(quasi matter)为主要引力源”。作为一个观察事实(当今宇宙平均物态系数为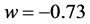 [64] 或者
[64] 或者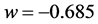 [65] ,可以取平均值为
[65] ,可以取平均值为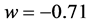 或者
或者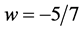 ),普通重子物质密度演化规律为
),普通重子物质密度演化规律为 ,其中宇宙标度因子(宇宙半径)
,其中宇宙标度因子(宇宙半径)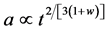 ,物质密度减弱得确实比较快,因而其远远小于准物质(quasi matter)密度
,物质密度减弱得确实比较快,因而其远远小于准物质(quasi matter)密度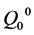 是有可能的,尽管过去(几十亿年前)宇宙减速膨胀时物质占主要。下面再较为具体地阐明这个问题:
是有可能的,尽管过去(几十亿年前)宇宙减速膨胀时物质占主要。下面再较为具体地阐明这个问题:
五维宇宙模型[63] 在当准物质(quasi matter)为主时,宇宙标度因子可以有一个精确的解析解 (其中
(其中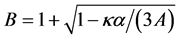 ,
,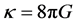 ),准(流体)物质密度
),准(流体)物质密度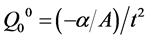 [63] 。这个解来自于五维宇宙的Friedmann方程,自然不满足四维宇宙的Friedmann方程。但是我们认为真实的宇宙是五维,而四维宇宙内的宇宙学观察结果要由五维宇宙模型来解释。而目前的观察宇宙学(observational cosmology)结果是结合四维宇宙的Friedmann方程来分析的,为了将五维宇宙模型的理论结果与观察宇宙学结果做比较,我们需要将五维宇宙的解析解代入四维宇宙的Friedmann方程 (即将四维宇宙的Friedmann方程所包含的参数结果当作我们的观察宇宙学的“实验结果”来看待),我们就可以得到四维膜宇宙内的等效物态系数(该物态就是上面所述的准流体)
[63] 。这个解来自于五维宇宙的Friedmann方程,自然不满足四维宇宙的Friedmann方程。但是我们认为真实的宇宙是五维,而四维宇宙内的宇宙学观察结果要由五维宇宙模型来解释。而目前的观察宇宙学(observational cosmology)结果是结合四维宇宙的Friedmann方程来分析的,为了将五维宇宙模型的理论结果与观察宇宙学结果做比较,我们需要将五维宇宙的解析解代入四维宇宙的Friedmann方程 (即将四维宇宙的Friedmann方程所包含的参数结果当作我们的观察宇宙学的“实验结果”来看待),我们就可以得到四维膜宇宙内的等效物态系数(该物态就是上面所述的准流体)  [63] 。如果等效物态系数为当今观察到的
[63] 。如果等效物态系数为当今观察到的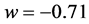 [64] [65] ,那么我们可以得到五维宇宙模型中的无量纲数值
[64] [65] ,那么我们可以得到五维宇宙模型中的无量纲数值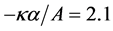 。为了保证上述五维宇宙模型中的解析解成立,需要让
。为了保证上述五维宇宙模型中的解析解成立,需要让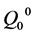 远大于普通重子物质密度(以及大于暗物质密度,如果它存在的话),即让这个“工作假设”成立。实际上,也只有在这个条件下,才可以得到五维宇宙模型(包含准流体方程
远大于普通重子物质密度(以及大于暗物质密度,如果它存在的话),即让这个“工作假设”成立。实际上,也只有在这个条件下,才可以得到五维宇宙模型(包含准流体方程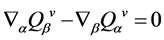 及五维宇宙的Friedmann方程)的解析解[63] 。根据四维宇宙中的Friedmann方程,我们有
及五维宇宙的Friedmann方程)的解析解[63] 。根据四维宇宙中的Friedmann方程,我们有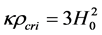 (
(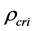 为宇宙临界密度)。当今观察宇宙学(observational cosmology)表明,重子物质与暗物质密度约占宇宙临界密度的30%[64] [65] ,故而我们要求
为宇宙临界密度)。当今观察宇宙学(observational cosmology)表明,重子物质与暗物质密度约占宇宙临界密度的30%[64] [65] ,故而我们要求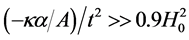 ,也即
,也即 。由于当今宇宙从减速膨胀过渡到加速膨胀,并非主要受单一物态影响,故而
。由于当今宇宙从减速膨胀过渡到加速膨胀,并非主要受单一物态影响,故而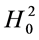 与
与 的关系比较复杂。如果宇宙成份是以零压物态为主,那么
的关系比较复杂。如果宇宙成份是以零压物态为主,那么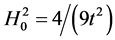 (根据四维宇宙中的Friedmann方程),实际因为宇宙加速膨胀,
(根据四维宇宙中的Friedmann方程),实际因为宇宙加速膨胀,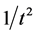 的系数肯定要比4/9大,且也与时间
的系数肯定要比4/9大,且也与时间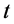 有关。为了简化问题,我们姑且认为
有关。为了简化问题,我们姑且认为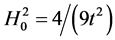 ,于是前述
,于是前述 条件,也即要求
条件,也即要求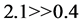 。这一条件显然可以成立(但不算很理想地成立)。如果暗物质不存在(所谓的暗物质效应其实来自第五维度贡献),普通重子物质仅仅占临界密度的3%到5%[64] [65] ,那么这一条件(五维宇宙以单一物态
。这一条件显然可以成立(但不算很理想地成立)。如果暗物质不存在(所谓的暗物质效应其实来自第五维度贡献),普通重子物质仅仅占临界密度的3%到5%[64] [65] ,那么这一条件(五维宇宙以单一物态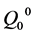 为主)就变为
为主)就变为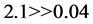 。这个条件显然很好地成立。所以,如果暗物质不存在,目前所得到的五维宇宙解析解[63] 已经足够可用。但由于暗物质可能确实存在(如关于星系暗物质的证据有很多),那么目前所得到的五维宇宙解析解就不那么精确,需要将四维宇宙中的暗物质考虑进去,就五维宇宙模型做更为复杂的计算。五维宇宙模型的功能在于不再需要假设暗能量的存在,仅仅准物质
。这个条件显然很好地成立。所以,如果暗物质不存在,目前所得到的五维宇宙解析解[63] 已经足够可用。但由于暗物质可能确实存在(如关于星系暗物质的证据有很多),那么目前所得到的五维宇宙解析解就不那么精确,需要将四维宇宙中的暗物质考虑进去,就五维宇宙模型做更为复杂的计算。五维宇宙模型的功能在于不再需要假设暗能量的存在,仅仅准物质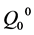 (它是引力规范理论的产物)以及第五维度就可以提供四维宇宙中所观察到的暗能量效应(以及部分暗物质效应),或者也可以说,它能比较自然地解释当今观察到的四维宇宙中的平均物态系数与减速参数。宇宙密度的偶遇(一致)问题(cosmic coincidence problem)也就不成为很麻烦的问题。
(它是引力规范理论的产物)以及第五维度就可以提供四维宇宙中所观察到的暗能量效应(以及部分暗物质效应),或者也可以说,它能比较自然地解释当今观察到的四维宇宙中的平均物态系数与减速参数。宇宙密度的偶遇(一致)问题(cosmic coincidence problem)也就不成为很麻烦的问题。
对于暗物质的讨论,要分星系暗物质和宇宙暗物质两种情形进行分析。后者是前者的总和。前者在星系尺度分布不均匀,后者在宇宙学尺度上可以看作呈现高度均匀与各向同性分布。上面的五维宇宙模型[63]并没有排除宇宙暗物质的存在。它至少证明,选择适当的准流体密度 ,五维宇宙模型可以提供与观察一致的平均物态系数(进一步得到与观察一致的减速系数);第五维度与准流体密度
,五维宇宙模型可以提供与观察一致的平均物态系数(进一步得到与观察一致的减速系数);第五维度与准流体密度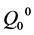 一起,可以提供一些暗物质与暗能量的效应[63] 。在五维宇宙模型中,暗能量与
一起,可以提供一些暗物质与暗能量的效应[63] 。在五维宇宙模型中,暗能量与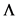 是可以不必直接引入的。但是暗物质是否一定需要,五维宇宙模型无法回答。如果暗物质真的存在,那么已经得到的五维宇宙模型的精确解析解[63] (这个解以准物质(quasi matter)为引力源,其能量–动量张量
是可以不必直接引入的。但是暗物质是否一定需要,五维宇宙模型无法回答。如果暗物质真的存在,那么已经得到的五维宇宙模型的精确解析解[63] (这个解以准物质(quasi matter)为引力源,其能量–动量张量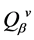 遵守公式(20)
遵守公式(20)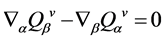 )就不再精确有效。由于五维宇宙模型已经可以包容与观察一致的平均物态系数,宇宙暗物质可以并不存在。那么这也就要求星系暗物质并不存在。在没有暗物质支撑下,五维模型是否也可以解释星系转动力学(主要是平坦转动曲线与Tully-Fisher关系)呢?有关星系平坦转动曲线与Tully-Fisher关系文献有很多,这里提供少许文献[66] (读者可以根据该文献中所引用的信息获得进一步资料)。五维模型确实有可能解释星系平坦转动曲线与Tully-Fisher关系。Wesson等人曾经利用五维空时空方程
)就不再精确有效。由于五维宇宙模型已经可以包容与观察一致的平均物态系数,宇宙暗物质可以并不存在。那么这也就要求星系暗物质并不存在。在没有暗物质支撑下,五维模型是否也可以解释星系转动力学(主要是平坦转动曲线与Tully-Fisher关系)呢?有关星系平坦转动曲线与Tully-Fisher关系文献有很多,这里提供少许文献[66] (读者可以根据该文献中所引用的信息获得进一步资料)。五维模型确实有可能解释星系平坦转动曲线与Tully-Fisher关系。Wesson等人曾经利用五维空时空方程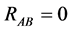 ,得到一个度规解[67] [68] ,其分量
,得到一个度规解[67] [68] ,其分量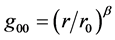 ,其中参量
,其中参量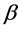 很小,故而
很小,故而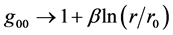 ,产生了引力势
,产生了引力势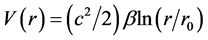 。因此,这样的引力势显然可以导致平坦转动曲线。但我们在这里需要特别指出的是,这样的引力势还可以解释Tully-Fisher关系。首先,我们要定出参数
。因此,这样的引力势显然可以导致平坦转动曲线。但我们在这里需要特别指出的是,这样的引力势还可以解释Tully-Fisher关系。首先,我们要定出参数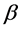 。我们知道,根据有关星系转动力学的观察事实[69] ,诸星系有一个共用的常数加速度参量
。我们知道,根据有关星系转动力学的观察事实[69] ,诸星系有一个共用的常数加速度参量 (根据对九个已经有良好测量的星系的转动曲线的分析可知这个常数加速度参量数值为
(根据对九个已经有良好测量的星系的转动曲线的分析可知这个常数加速度参量数值为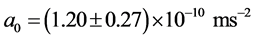 [69] )。利用该常数加速度
[69] )。利用该常数加速度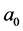 ,我们可以定义一个星系特征长度
,我们可以定义一个星系特征长度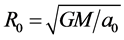 ,
,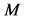 为星系可见质量(由重子物质构成,如恒星、尘埃与气体等)。另外,尤其要说明的是,Wesson等得到的度规分量
为星系可见质量(由重子物质构成,如恒星、尘埃与气体等)。另外,尤其要说明的是,Wesson等得到的度规分量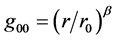 针对五维真空引力(即无源),也就是说,它仅仅在当星系盘上径向坐标
针对五维真空引力(即无源),也就是说,它仅仅在当星系盘上径向坐标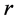 远大于其特征长度
远大于其特征长度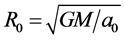 时才适用;如果径向坐标
时才适用;如果径向坐标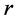 远小于其特征长度,那么星系可见质量
远小于其特征长度,那么星系可见质量 就要起着作用,星系引力势就退化为牛顿引力势,Wesson的结果就不再适用。
就要起着作用,星系引力势就退化为牛顿引力势,Wesson的结果就不再适用。
因此在当径向坐标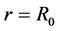 时,牛顿引力
时,牛顿引力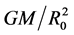 与Wesson引力
与Wesson引力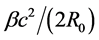 都将等于
都将等于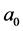 (在
(在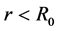 时,牛顿引力占上风;在
时,牛顿引力占上风;在 时,Wesson引力占上风;在
时,Wesson引力占上风;在 ,两者相等,都等于
,两者相等,都等于 )。于是我们可以定出,
)。于是我们可以定出,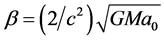 。度规
。度规 中的
中的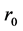 取值为多少,倒不影响对Tully-Fisher关系的解释,但是我们可以令
取值为多少,倒不影响对Tully-Fisher关系的解释,但是我们可以令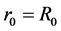 ,这样可以有一个好处,在当径向坐标
,这样可以有一个好处,在当径向坐标 时,
时,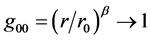 (为牛顿引力与Wesson引力的分界线)。这样,在星系大尺度上,在Wesson引力作用下,星系转动曲线方程将是
(为牛顿引力与Wesson引力的分界线)。这样,在星系大尺度上,在Wesson引力作用下,星系转动曲线方程将是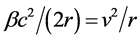 ,代
,代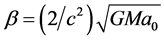 入该式,星系转动线速度的平方
入该式,星系转动线速度的平方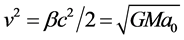 。星系转动线速度的平方与星系可见质量
。星系转动线速度的平方与星系可见质量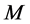 的平方根成正比,这就是Tully-Fisher关系。需要指出的是,上面将星系质量看作球对称分布,并未看作为圆盘分布。圆盘引力非常复杂,但幸好包括银河系在内的诸多星系,多数可见重子物质分布在小于4万光年的半径内(可为球状分布),但是其星系转动曲线的平坦性却一直延伸到半径为8万光年且没有一丝下降的趋势(也即有可能在半径为10~12万光年范围,还维持着平坦转动曲线)。如此一来,在半径4到12万光年范围看来,其引力源主要为以银心为中心、在小于4万光年的半径内分布着的可见重子质量
的平方根成正比,这就是Tully-Fisher关系。需要指出的是,上面将星系质量看作球对称分布,并未看作为圆盘分布。圆盘引力非常复杂,但幸好包括银河系在内的诸多星系,多数可见重子物质分布在小于4万光年的半径内(可为球状分布),但是其星系转动曲线的平坦性却一直延伸到半径为8万光年且没有一丝下降的趋势(也即有可能在半径为10~12万光年范围,还维持着平坦转动曲线)。如此一来,在半径4到12万光年范围看来,其引力源主要为以银心为中心、在小于4万光年的半径内分布着的可见重子质量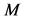 。圆盘模型似不需要考虑。
。圆盘模型似不需要考虑。
Tully-Fisher关系显示星系转动速度只与星系可见质量(普通重子物质)有关,与所谓的“暗物质”无关。当然,普通重子物质的引力场如果是牛顿引力场,则无法解释平坦转动曲线与Tully-Fisher关系。这要求或者引力定律、或者基本力学定律需要在星系尺度上修改。在当前对星系平坦转动曲线的研究中,一般有三条路子:①失踪质量,即冷暗物质;②修改(modified)或者扩展(extended)的的引力理论,如高阶导数理论(在Einstein-Hilbert作用量中添加一些曲率平方项等)、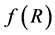 引力、Lovelock引力等;③修正的牛顿动力学(MOND),即认为牛顿第二定律
引力、Lovelock引力等;③修正的牛顿动力学(MOND),即认为牛顿第二定律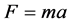 在低加速度时不再成立,而是变为
在低加速度时不再成立,而是变为 。第①种方案不修改任何引力理论,只是要求有超越粒子物理标准模型的基本粒子(如最轻超对称粒子)[70] ;第②种方案修改了爱因斯坦引力理论;第③种方案修改了(经典粒子)力学。但上面的五维模型没有修改力学,也保留了爱因斯坦引力场方程、没有引入暗物质,只引入了第五维,因此可能是最自然、最经济的方案。
。第①种方案不修改任何引力理论,只是要求有超越粒子物理标准模型的基本粒子(如最轻超对称粒子)[70] ;第②种方案修改了爱因斯坦引力理论;第③种方案修改了(经典粒子)力学。但上面的五维模型没有修改力学,也保留了爱因斯坦引力场方程、没有引入暗物质,只引入了第五维,因此可能是最自然、最经济的方案。
8. 结论
本文综述了引力规范理论的研究简史、一个最新的引力规范理论及其特点,研究了其在宇宙学与天体物理中的应用。从电磁场的Abel规范相互作用理论到Yang-Mills的非Abel规范相互作用理论,及其在基本粒子标准模型中的成功,暗示要求引力最好也是一种Yang-Mills规范相互作用,而爱因斯坦引力理论离Yang-Mills方程形式相去甚远。由于包括电磁真空能在内的量子真空能具有几近发散的能量密度,在爱因斯坦引力场方程中出现了一个重要麻烦,即存在一个巨大的疑问:理论上所要求的几近无穷大的量子真空能密度导致的巨大引力场(以及其巨大的引力落差即潮汐力)为何在宇宙学观察中没有显现?我们将自旋仿射联络看作引力规范场,建立了一个局域Lorentz群规范理论,该理论不再出现真空能引力问题,且在高能情形下,有量纲的引力常数被无量纲的引力常数取代[3] [51] -[54] 。为了解决真空能引力与真空能宇宙常数问题,文献中提出了各种方案[13] -[16] 。近来一个典型的理论是Moffat等人的非局域量子引力理论[71] ,其在Einstein-Hilbert作用量与普通物质场作用量中插入了一些偏导数算符的指数函数项(形如 等),以期待引入一个低能标(如
等),以期待引入一个低能标(如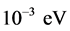 ),让量子真空能密度为
),让量子真空能密度为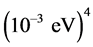 ,从而接近目前的宇宙临界密度,将此量子真空能作为宇宙加速膨胀之源[71] 。我们认为这类理论对于解决真空能引力与真空能宇宙常数问题,思路都过于直接且单纯。况且,
,从而接近目前的宇宙临界密度,将此量子真空能作为宇宙加速膨胀之源[71] 。我们认为这类理论对于解决真空能引力与真空能宇宙常数问题,思路都过于直接且单纯。况且,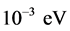 的真空能能标其对应的截断波长为
的真空能能标其对应的截断波长为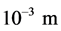 (截断频率为
(截断频率为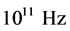 ),一般的金属微腔实验均可以轻易否定它。本文所介绍的引力规范理论不但把无穷大的真空能引力效应消去了,还产生了一个等效宇宙“常数”(既可以是变的、也可以是常数),原本(按照常例)可以将它作为宇宙加速膨胀之源,但是我们还是放弃了这种惯常做法(因为否则这会导致宇宙密度的cosmic coincidence问题)。我们利用五维宇宙模型(以及由引力规范理论导致的准流体)来解释宇宙加速膨胀。因此,该理论包含了丰富的物理因素。
),一般的金属微腔实验均可以轻易否定它。本文所介绍的引力规范理论不但把无穷大的真空能引力效应消去了,还产生了一个等效宇宙“常数”(既可以是变的、也可以是常数),原本(按照常例)可以将它作为宇宙加速膨胀之源,但是我们还是放弃了这种惯常做法(因为否则这会导致宇宙密度的cosmic coincidence问题)。我们利用五维宇宙模型(以及由引力规范理论导致的准流体)来解释宇宙加速膨胀。因此,该理论包含了丰富的物理因素。
该引力规范理论中出现了一种准流体的“能量-动量张量”,它不但提供了一个宇宙学常数,且还提供了一种具有辐射特性的准流体(暗辐射、热暗物质)。本文只考虑了标量物质场(引力源) [3] [51] -[54] 。对于引力源为矢量场和旋量场的情形,亦值得考虑,这要求引入挠率,推广该理论,如至少还要引入时空平移规范对称(spacetime translational gauge symmetry)的引力。时空平移规范对称引力理论已经有多家[45] [72] [73] 。这类理论将导数算符 作为时空平移规范群生成元,建立非Abel规范理论。既有在弯曲时空[45] 、也有在平直时空[72] -[74] 建立“平移规范对称”的引力规范理论(例如本人基于平移规范对称性得到的平直时空引力场张量[75] 为
作为时空平移规范群生成元,建立非Abel规范理论。既有在弯曲时空[45] 、也有在平直时空[72] -[74] 建立“平移规范对称”的引力规范理论(例如本人基于平移规范对称性得到的平直时空引力场张量[75] 为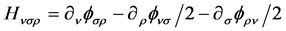 与Zhang等人的结果[73] 一致,
与Zhang等人的结果[73] 一致,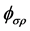 即为Hsu等人的引力规范势[72] )。但是,这类平直时空引力规范理论往往无法解释水星近日点进动值(不是偏大就是偏小)。
即为Hsu等人的引力规范势[72] )。但是,这类平直时空引力规范理论往往无法解释水星近日点进动值(不是偏大就是偏小)。
在当引力源为矢量物质场和旋量物质场时,在引力规范理论中,也需要引入具有矢量和旋量特性的重伴随场 。为了保证等效原理成立(如引力常数
。为了保证等效原理成立(如引力常数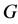 具有普适性),要求具有矢量和旋量特性的重伴随场的质量
具有普适性),要求具有矢量和旋量特性的重伴随场的质量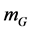 也与上述标量重伴随场
也与上述标量重伴随场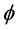 的质量
的质量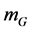 相等。在低能情形(远低于普朗克能标),这本身是奇怪的(因为我们知道实际基本粒子的质量数值分布随机),但在高能时(如普朗克能标附近),由于超对称的存在,让具有矢量特性和旋量特性的重伴随场的质量
相等。在低能情形(远低于普朗克能标),这本身是奇怪的(因为我们知道实际基本粒子的质量数值分布随机),但在高能时(如普朗克能标附近),由于超对称的存在,让具有矢量特性和旋量特性的重伴随场的质量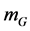 也与标量重伴随场的质量
也与标量重伴随场的质量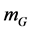 相等,这是一个自然的结论了。因而我们认为,该引力理论[3] [51] -[54] 其实暗示了超对称在筑构新引力理论时确实是必要的,否则就无法解释引力常数
相等,这是一个自然的结论了。因而我们认为,该引力理论[3] [51] -[54] 其实暗示了超对称在筑构新引力理论时确实是必要的,否则就无法解释引力常数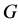 的普适性。
的普适性。
致 谢
本文原稿曾提交给全国高校第十三届《电动力学》研讨会(哈尔滨,2011年8月7日~11日)交流,特此致谢。感谢Dr. Zhi-Yuan Shen推荐Hsu, J.-P.与Hsu, L.的专著[72] 于我。