1. 引言
传染病已经严重的威胁了人类的健康和生命。根据世界卫生组织的调查,世界上近三分之一的人口死于传染病,这对人类来说是个可怕的数字。为了预防和控制传染病的传播,许多学者运用数学模型来研究传染病传播的动力学行为 [1] [2] [3]。传统的传染病模型将整个人群分为三类:易感人群S,受感染的人群I和康复人群R。对于某些疾病,一些从感染中恢复的人对再次感染有短期或长期的免疫力。因此,我们必须在模型中考虑免疫效应,以便更好地反映传染病传播的实际动态,及时预测未来传染病的爆发。免疫可以在一个人从感染中恢复后自然获得,也可以通过母亲的抗体传染给新生儿。由于一些疾病提供终身免疫,而另一些疾病只提供临时免疫,因此免疫的持续时间各不相同。
时滞微分方程已成功地应用于SIR、SIS和SIRS流行病模型。Hethcote和van den Driessche [4] 考虑了一种SIS传染病的模型,该模型具有可变的种群规模和恒定的感染持续时间。研究还得到Hopf分岔可能发生在特有的平衡点上,并导致某些参数值的周期性振荡。Beretta et al. [5] 研究了具有分布时滞的SIR流行病模型的全局稳定性,并且描述了个体失去传染性所需的时间。使用了李亚普诺夫函数来确认无病和地方病平衡点的全局稳定性。最近,Brauer et al. [6] 应用线性发病率研究感染性疾病在双菌斑环境中的传播动态。主要的假设有一个固定的临时免疫期,在此期间恢复的人群返回到易感人群中。Konstantin et al. [7] 主要认为免疫力随着时间的推移而减弱,提出了一种考虑免疫周期变化的分布式时滞微分方程模型,并且证明了无病平衡和地方病平衡的稳定性。这意味着在一个人康复之后,只有在对这种疾病有了一段时间的免疫力之后才会再次变得易感。模型如下:
 (1)
(1)
这里的所有参数均为正的常数,
代表易感者的数量,
代表染病者的数量,
代表恢复者的数量。
表示招募率,
代表总人口,
表示自然死亡率,
表示传染率系数,
表示恢复率并且有
。
另一方面,由于传染病在传播过程中受到环境噪声的影响 [8] - [13]。确定性数学微分方程模型在预测传染病传播动态方面具有一定的局限性,因此,在数学模型中考虑随机噪声是必要的。与确定性模型相比,随机微分方程模型能更准确地预测未来传染病的传播。目前,引入随机扰动的方法很多 [14] - [20]。一般情况下,环境波动可以用高斯白噪声来模拟。在这篇论文中,受文献 [21] 的启发,假设
在平均值附近波动,所以
可以看作是这样一个随机变量
。因此,随机微分方程描述如下:
(2)
是独立的标准布朗运动, 表示标准高斯白噪声强度。由于系统(2)中的前两个方程与第三个方程无关,所以系统可以等价地写成:
表示标准高斯白噪声强度。由于系统(2)中的前两个方程与第三个方程无关,所以系统可以等价地写成:
(3)
2. 全局正解的存在唯一性
引理2.1 任何初始值
和
对于
有
。当
时,系统(3)存在唯一的正解
且该解以1为概率几乎处处。
证明:因为系统(3)的系数满足局部Lipschitz条件,因此,对于任意给定的初值
,系统(3) 存在唯一的局部解
,
,其中
表示爆破时间 [22]。为了证明
是全局的,我们只需要证明
几乎处处成立。令
是一个足够大的数使得
位于区间
中。对每一个整数
,定义停时
在这篇文章中,记
(通常
表示空集)。明显,当
时,
是单调递增的。令
,
有
几乎处处。因此我们只需要证明
几乎处处。利用反证法,假设
,则存在常数
和 使得
使得
因此,存在一个整数
使得
(4)
定义
-函数
(5)
给系统(3)应用Itô’s公式,对于所有的
时有
。也可以得到
由此得出
(6)
其中
对(6)两边从0到t积分,得到
(7)
因为
等于0,所以可以得到
对于(7),令
,可以得到
,这就产生了矛盾。
因此有
a.s.因此,上述证明就完成了。
引理2.2
是模型(3)的解,
和
对于所有的
有
是解的初值,则有
(8)
证明:
(9)
首先需要证明
用假设方法来证明
因此,我们得到
因此,有
和
满足
存在一个足够大的常数M使得
,当
时有
显然,当
有
因此,
令
,可以得到
(10)
此外,当
,我们可以得到以下方程
因此,可以得到
进一步,可以得到
这就产生了矛盾,因为上面不等式的右边是有限的。于是,我们得到
(11)
由于
和
是连续的,结合(9)有
因此,我们完成了上述证明。
显然,我们可以由引理2.1和引理2.2得到以下定理:
定理2.3 令
是系统(3)带有任意初值
的解,那么系统(3)存在唯一的全局解
,且该解以概率1停留在
中,即当
时,系统(3)的解
。
3. 疾病的灭绝性
在这一节中,我们将讨论疾病的灭绝,定义
定理3.1 令
是系统(3)的解,其中
和
对于
有
是解的初值,则
1) 如果
,则
2) 如果
,则
证明:根据文献 [23] 和定理2.1,当所有的数
时,对于任意的数
,存在一个常数
满足
,应用Itô’s公式,其中
,那么可以得到
(12)
先证明上述定理的结论(2)。如果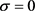 ,可以得到
,可以得到
对于所有的
,显然有
由于
的任意性,可以得到
根据方程(12),如果
,那么对于任意的
有
接下来构造一个函数
当
时,
是单调递增函数,当
时,
是单调递减函数。根据条件
就可以得到
。因此,选择一个常数
使得
和
。 对于所有的
,因为
,所以有
因此,如果
时有
(13)
根据强大数定理有
根据(13)可以得到
由于
和
的任意性,可以得到
接下来证明定理3.1的结论(1)。如果
,则
在
时有一个最大值
。因此,对所有的
时有
然后
与上述方法相似,我们还可以得到
因此,上述证明已完成。
注3.2 由定理3.1表明,如果条件(1)
或者(2)
和
满足,则对于系统(3)的任意一个解
总是有
。这意味着疾病灭绝。
4. 结论
本文研究了具有分布时滞的参数扰动下的SIRS传染病模型,并证明了系统(3)解的唯一性和存在性,
并且通过构造合适的李雅普诺夫函数得到疾病灭绝的条件,即
或者
和
满足时
疾病灭绝。
另一方面,本文考虑的内容还不全面,还有许多问题值得进一步的考虑,例如,我们只考虑由白噪声描述的连续随机扰动。事实上,还有一些随机扰动可以用电报噪声和Lévy噪声来模拟。我们只考虑了疾病灭绝和持续的充分条件,疾病灭绝和持续的必要条件并没有得到。本文所考虑的发生率是双线性和标准发生率,今后的工作还可以研究其他形式发生率(如饱和发生率)下传染病模型的稳定性问题。