摘要: 本文主要考虑具有对数非线性项的分数阶阻尼波动方程
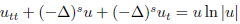
的初边值 问题,其中s ∈ (0, 1)。 算子(−∆)
s为分数阶Laplace算子,近年来,该算子成为了物理学、 金融数 学、 流体动力学等学科领域中的研究热点。 本文在任意初始能量下,利用Galerkin逼近法和压缩
映射原理,证明该方程解的局部适定性。
Abstract:
In this paper, we mainly deal with the initial-boundary value problem for the frac- tional damped wave equations
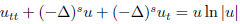
, where s ∈ (0, 1). The operator (−∆)
s is the fractional Laplace operator. In recent years, this operator has
become a research hotspot in physics, financial mathematics, fluid dynamics and oth- er disciplines. At the arbitrary initial energy levels, the local well-posedness of weak solutions to above problem is proved by using Galerkin approximation method and contraction mapping principle under some certain conditions.