摘要: 本文根据n维耦合谐振子矩阵形式的薛定谔(Schrödinger)方程,在表象理论的基础上,得到了哈密顿(Hamiltonian)算子ˆH 的矩阵元Hnn。通过构建一类完备的赋范线性空间,由泛函理论,证明了Hnn在此空间中是有界算子,同时求得哈密顿算子的本征值E;进而利用矩阵理论得到E的谱条件数公式。从这个表达式出发,得到了能量E的算子范数与谐振子的状态
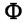
之间的关系式;研究了E的谱条件数与算子范数之间的关系,并估算E的算子范数上、下界的值,给出了能量谱条件数值大小的原因。结果表明:求出谱条件数与算子范数的大致范围,就可以根据谱条件数的准确值,在表象理论框架内,估测谐振子两个状态之间的差异程度,分析谐振子的状态特征。
Abstract:
This paper according to the matrix form of Schrödinger equation of n-dimension coupled harmonic oscillator, on the basis of representation theory, the element of matrix Hnn of Hamiltonian operator ˆH is derived. Through con-structing one of complete normed linear space, the Hnn is proved to be boundedness in this space by using functional theory, and eigenvalue E of Hamiltonian operator is obtained; and then the author get the spectrum condition number formula of E that is made use of matrix theory. From this expressions, the formula of operator norm of energy E and harmonic oscillator’s
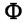
state is acquired. Researching the relationship between spectrum condition number of E and operator norm, the supremum and infimum of E operator norm is estimated, the reason of numerical size of energy spectrum condition number is presented. It turned out that: when the approximately range of spectrum condition number and operator norm is achieved, under the representation theory frame, the difference degree between two states of har-monic oscillator are estimated, which in terms of the exactly value of spectrum condition number, and analysis the fea-ture states of harmonic oscillator.