一类二阶半正Dirichlet边值问题正解的存在性和多解性
Existence and Multiplicity of PositiveSolutions for a Class of Second-OrderDirichlet Boundary Value Problems
DOI: 10.12677/PM.2023.137206, PDF,    国家自然科学基金支持
作者: 李存丽：西北师范大学，数学与统计学院，甘肃 兰州
关键词: 正解；半正问题；不动点指数理论；上下解；Positive Solutions； Semi-Positone Problem； Fixed Point Index； Upper and Lower Solutions
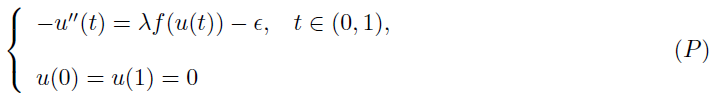
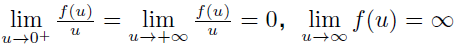
摘要: 考察二阶半正 Dirichlet 边值问题 正解的存在性与多解性,其中入为正参数，f∈C([0,∞),[0,∞))，存在∧> 0，使得∈∈[0,∧]。当f 满足时，运用不动点指数理论和上下解方法证明了存在常数λ∗ > 0，使得当λ>λ∗时，问题(P) 至少存在两个正解。
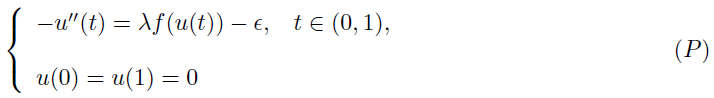
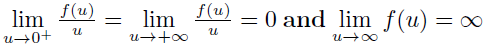
Abstract: In this paper,we are considered with the existence and multiplicity of positive solutions for second-order Dirichlet boundary value problems where λ is a positive parameter, f∈C([0,∞),[0,∞)), there exists ∧> 0, such that ∈∈[0,∧]. When f satisfies , we apply a fixed point index theorem and the method of the upper and lower solutions to prove that there exists λ∗ > 0 such that the problem (P) has at least two positive solutions for λ>λ∗.
文章引用：李存丽. 一类二阶半正Dirichlet边值问题正解的存在性和多解性[J]. 理论数学, 2023, 13(7): 2007-2016. https://doi.org/10.12677/PM.2023.137206

参考文献

[1] Erbe, L.H. and Wang, H.Y. (1994) On the Existence of Positive Solutions of Ordinary Differential Equations. Proceedings of the American Mathematical Society, 120, 743-748. [Google Scholar] [CrossRef] 
[2] Li, Y.X. (2003) Positive Solutions of Second-Order Boundary Value Problems with Sign- Changing Nonlinear Terms. Journal of Mathematical Analysis and Applications, 282, 232-240.[CrossRef] 
[3] Efendiev, B.I. (2018) The Dirichlet Problem for an Ordinary Continuous Second-Order Differential Equation. Matematicheskie Zametki, 103, 295-302. [Google Scholar] [CrossRef] 
[4] Zhao, J. and Wang, Y.C. (2017) Nontrivial Solutions of Second-Order Singular Dirichlet Systems. Boundary Value Problems, 2017, Article No. 180. [Google Scholar] [CrossRef] 
[5] Alkhutov, Y. and Borsuk, M. (2017) The Dirichlet Problem in a Cone for Second-Order Elliptic Quasi-Linear Equation with The p-Laplacian. Journal of Mathematical Analysis and Applications, 449, 1351-1367. [Google Scholar] [CrossRef] 
[6] Gritsans, A., Sadyrbaev, F. and Yermachenko, I. (2016) Dirichlet Boundary Value Problem for the Second-Order Asymptotically Linear System. International Journal of Differential Equations, 2016, Article ID: 5676217. [Google Scholar] [CrossRef] 
[7] Wan, H.T. (2015) The Second-Order Expansion of Solutions to a Singular Dirichlet Boundary Value Problem. Journal of Mathematical Analysis and Applications, 427, 140-170. [Google Scholar] [CrossRef] 
[8] Dumanyan, V.Z. (2014) On the Solvability of the Dirichlet Problem for a Second-Order Elliptic Equation. Doklady. Natsional'naya Akademiva Nauk Armenii, 114, 295-308.
[9] Zhou, J.W. and Li, Y.K. (2009) Existence and Multiplicity of Solutions for Some Dirichlet Problems with Impulsive Effects. Nonlinear Analysis: Theory, Methods Applications, 71, 2856-2865. [Google Scholar] [CrossRef] 
[10] Castro, A. and Shivaji, R. (1988) Non-Negative Solutions for a Class of Non-Positone Problems. Proceedings of the Royal Society of Edinburgh, 108, 291-302. [Google Scholar] [CrossRef] 
[11] Lions, P.L. (1982) On the Existence of Positive Solutions of Semilinear Elliptic Equations. Society of Industry and Applied Mathematics, 24, 441-467. [Google Scholar] [CrossRef] 
[12] Guo, D. and Lakshmikantham, V. (1988) Nonlinear Problems in Abstract Cones. Academic Press, Orlando, FL.
[13] Habets, P. and Zanolin, F. (1994) Upper and Lower Solutions for a Generalized Emden-Fowler Equation, Journal of Mathematics Analysis and Applications, 181, 684-700. [Google Scholar] [CrossRef] 
[14] Zhang, X. and Feng, M. (2019) Bifurcation Diagrams and Exact Multiplicity of Positive Solutions of One-Dimensional Prescribed Mean Curvature Equation in Minkowski Space. Communications in Contemporary Mathematics, 21, Article 1850003. [Google Scholar] [CrossRef] 
[15] Whyburn, G.T. (1958) Topological Analysis. Princeton Mathematical Series, No. 23. Princeton University Press, Princeton, NJ.

为你推荐