摘要: 本文研究了一类半正周期边值问题

正解的存在性,其中λ为正参数,ε是一个正数,a,b∈C(ℝ,[0,∞)) 是1-周期函数且∫
01a(t)dt > 0,∫
01b(t)dt > 0,f,g∈C([0,∞),[0,∞)),τ(t)是连续1-周期函数。运用上下解方法和拓扑度理论,得到存在常数λ∗ > 0,使得当λ∈(0,λ∗)时,问题(P)存在两个正解。
Abstract:
We are concerned with the existence of positive solutions for a class of semi-positive
periodic boundary problems
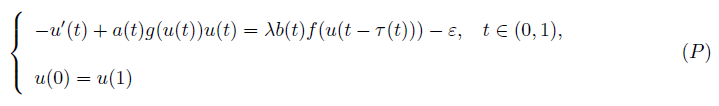
where λ is a positive parameter, ε is a positive constant,a,b∈C(ℝ,[0,∞)) is a 1-periodic function, ∫
01a(t)dt > 0,∫
01b(t)dt > 0. f,g∈C([0,∞),[0,∞)),τ(t) is a continuous 1-periodic function. By using the method of upper and lower solutions and topological degree theory, we show that there exists a constant λ∗ > 0, such that the problem (P) has two positive solutions for λ∈(0,λ∗).