1. 引言
1996年,Hide等提出了一个self-exciting Faraday圆盘单极发电机,它是一个非线性的三维动力系统 [1]。在此基础上,逐渐发展了很多改进的模型 [2] - [10],其中Hide和Moroz提出了一类四维的发电机,它描述了方位涡流(azimuthal eddy currents)的作用。其模型如下:
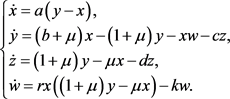 (1.1)
(1.1)
其中x和y分别表示圆盘和线圈的磁通量,z表示电动机电枢的角转速,w表示圆盘的角转速。
Moroz在文献 [11] 中已经做了一些数值分析去研究系统(1.1)的zero-zero-Hopf分支,然而并没有严格的证明。本文将给出系统存在zero-zero-Hopf分支的证明,并把相应的周期解求出来。
一个zero-Hopf或zero-zero-Hopf奇点意味着可能出现混沌。近年来,虽有一些文献研究三维混沌系统的zero-Hopf分支 [12] [13] [14],但由于高维系统的复杂性,对四维及以上系统的zero-zero-Hopf分支的研究非常少 [15]。Cid-Montiel等在文献 [15] 中研究了一个四维超混沌的Lorenz系统的zero-Hopf分支。Chen 等在文献 [16] 中研究了一个广义Lorenz-Stenflo超混沌系统zero-zero-Hopf分支。
2. 超混沌和zero-zero-Hopf分支
系统(1.1) 总有一个奇点
。当
时,还有如下的两个奇点:
,
其中
。
对初始值
和参数
,我们计算出来Lyapunov指数是0.2927,0.1826,0,−19.0777。因此系统是超混沌的,图1显示了一个超混沌吸引因子。
图1是系统(1.1)的超混沌吸引因子,其中初始值
,参数
。
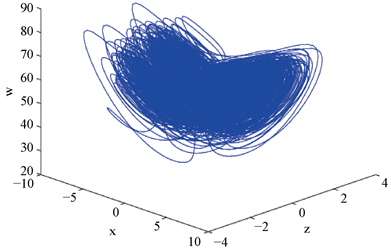
Figure 1. Hyperchaotic attraction factor of system (1.1)
图1. 系统(1.1)的超混沌吸引因子
下面我们应用平均理论去研究系统(1.1)的zero-zero-Hopf分支。当
,一些表达式过于庞大。为了表达的方便,我们令
。
理论1 对
,原点
是系统(1.1)的一个zero-zero-Hopf奇点。在
的特征值是0,0,
,其中
。
证明. 在
的特征方程是
(2.1)
当
时,(2.1)有根0,0,
,其中
。
利用平均理论,我们能证明下面的定理。
理论2 令
这里
。
假设
,那么系统(1.1)有一个zero-zero-Hopf分支。当
且
足够小时,在
产生两个周期解。如果
,那么这两个周期解是稳定的。
证明. 作变换
系统(1.1)变成
(2.2)
令
。我们把
又表示为
,那么系统(2.2)变为
(2.3)
下面利用平均理论来研究系统(2.2)的动力学行为。首先我们考虑下面这个未扰动系统的初值问题
(2.4)
系统(2.4)的解是
,其中
当
时,系统(2.4)的所有解
是周期的,其中周期为
。沿着一个周期解
,
其线性化系统
的基解矩阵
是
其中
计算积分
其中
解方程
,我们得到下面的解
对应奇点
。对其他两个解
和
,我们有
因此根据平均理论,系统(2.3)存在的两个周期为T的解
和
,当 时,有
和
。考虑到系统(2.2)和(2.3)的关系,系统(2.3)的两个周期解分别对应系统(2.2)的两个周期解
和
。
时,有
和
。考虑到系统(2.2)和(2.3)的关系,系统(2.3)的两个周期解分别对应系统(2.2)的两个周期解
和
。
最后,我们计算Jacobian矩阵
和
的特征值来判断两个周期解的稳定性。对这两个矩阵而言,特征多项式是相同的,如下
(2.5)
当
时,方程(2.5)的根都有负实部,这意味着在
产生的两个周期解
和
是稳定的。
3. 结束语
通过选取适当的参数,我们计算发现系统有两个正的Lyapunov指数,是一个超混沌的系统。不同于前人的数值工作,本文对系统存在zero-zero-Hopf分支给出了严格的证明,并给出了周期解的表达式,以及周期解稳定的条件。
NOTES
*通讯作者。