摘要:
设n为自然数,σ(n)表示n的所有正因子和函数。令d是n的真因子,若n满足σ(n)=2n-d ,则称n为亏因子为d的亏完全数。在参考文献的基础上,本文讨论了具有四个素因子的奇亏完全数的一些性质,证明了 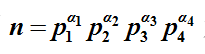 为具有四个不同素因子的奇亏完全数,则有p1 = 3, p2 ≤ 13。
为具有四个不同素因子的奇亏完全数,则有p1 = 3, p2 ≤ 13。
Abstract:
For a positive integer n, let σ(n) denote the sum of the positive divisors of n. Let d be a proper divisor of n, we call n a deficient-perfect number if
σ(n)=2n-d . On the basis of the references, we characterize some properties of odd deficient-perfect numbers with four distinct prime divisors. We prove that if
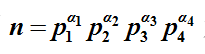
is an odd deficient-perfect number, then p
1 = 3, p
2 ≤ 13, and improve the result of the references.